
Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Example 8 (7.8) on =4+2n 6n2.Then on o(n2+6)for all >0. Remark If on =o(nA),then bn =O(nA).Intuitively,if on grows at a rate slower than n,it will grow at most at a rate ofn入. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 16/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 16/186 Example 8 (7.8) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Remark

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Lemma 1 (7.1) Let an and bn be scalars. (1)If an =O(n)and on =O(n#),then anon =O(nAtu),an+ bn=O(n),where k=max(入,u). (2)If an o(nA)and on o(n"),then anbn o(nAtu),an bn=o(n),where k=max(入,u). (3)Ifan=O(m入)and bn=o(n“,then anbn=o(nλ+),an+ bn=O(n),where K=max(入,u). Proof Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 17/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 17/186 Lemma 1 (7.1) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Proof
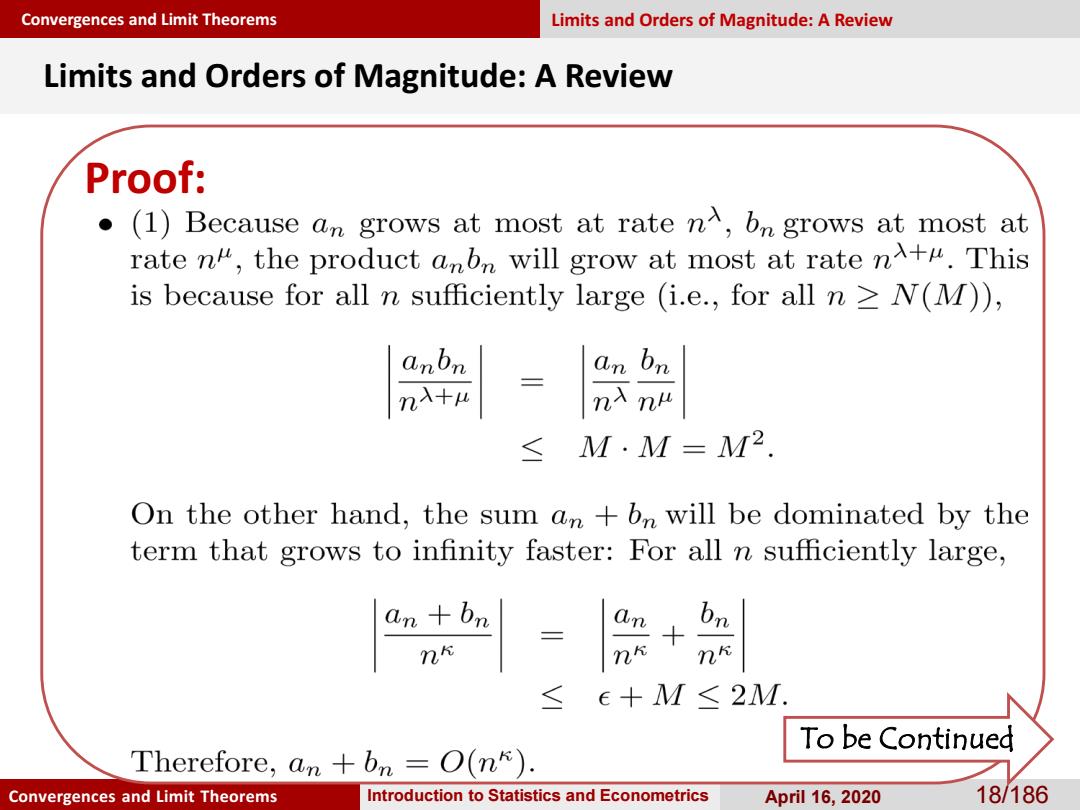
Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Proof: ●(1)Because an grows at most at rate n入,bn grows at most at rate n#,the product anon will grow at most at rate nA+#.This is because for all n sufficiently large (i.e.,for all n >N(M)), anbn An n n入+u n入 ≤ M.M=M2. On the other hand,the sum am +on will be dominated by the term that grows to infinity faster:For all n sufficiently large, =+ ≤e+M<2M. To be Continued Therefore,an +on-O(n). Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 18/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 18/186 Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Proof: To be Continued

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Proof: .(2)Similar to the proof of Result (1). .(3)The product anon =o(n+#)because anon an bn ≤M· bn 0asm→o given an=O(nλ)and bn=o(n“). Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 19/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 19/186 Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Proof:

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Example 9 (7.9) Suppose an =O(1),and bn=o(1).Then anbn o(1), an +bn=O(1). Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 20/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 20/186 Example 9 (7.9) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review