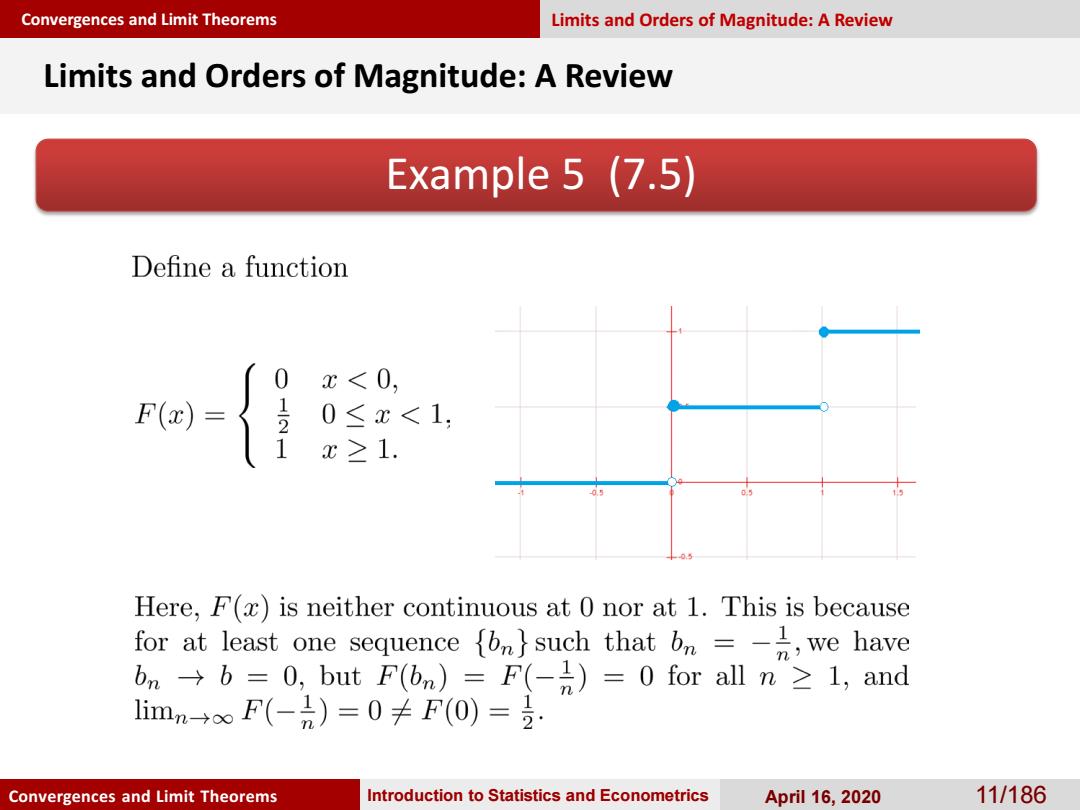
Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Example 5 (7.5) Define a function 如=之专一 x<0, 0≤x<1: x≥1. 05 Here,F(x)is neither continuous at 0 nor at 1.This is because for at least one sequence {on}such that on=-,we have bn→b=0,butF(bn)=F(-)=0 for all n≥1,and limn→oF(-)=0≠F(0)=. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 11/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 11/186 Example 5 (7.5) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review
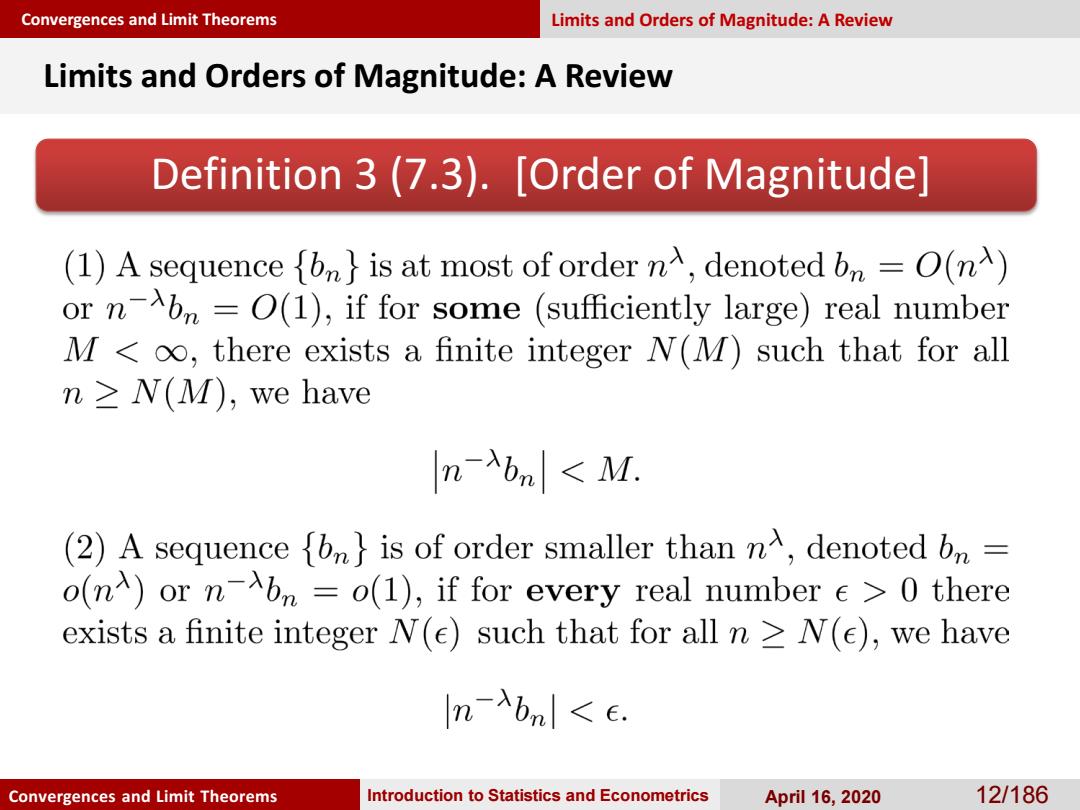
Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Definition 3 (7.3).[Order of Magnitude] (1)A sequence fon}is at most of order n,denoted n=O(nA) or n=O(1),if for some (sufficiently large)real number M <oo,there exists a finite integer N(M)such that for all m≥W(M),we have n-Aon M. (2)A sequence fon}is of order smaller than n^,denoted b o(nA)or nn=o(1),if for every real number e>0 there exists a finite integer N(e)such that for all nN(e),we have In-onl e. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 12/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 12/186 Definition 3 (7.3). [Order of Magnitude] Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Remarks: 。In the definition ofon=O(n入),the constant M is usu- ally set to be a very big number.Note that it suffices to find one constant M only. 。For入>0,bn=O(nλ)implies that on grows to infinity at a rate slower than or at most equal to n.In par- ticular,if limn=C<oo,then bn =O(nA)or n-Aon =O(1). Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 13/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 13/186 Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Remarks:

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Example 6 (7.6) on =4+2n+6n2.Then on =O(n2),because bn 4 2n 6m2 2 n2 n2 n2 6<2M=2.6(say) for all n sufficiently large.Intuitively,the order of bn is determined by the dominant term (i.e.,n2)that grows to infinity fastest. Remark ●It is possible that lim→obn/n入does not exist but|bn/nλl is bounded;in this case,we still have on=O(nA). Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 14/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 14/186 Remark Example 6 (7.6) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review

Convergences and Limit Theorems Limits and Orders of Magnitude:A Review Limits and Orders of Magnitude:A Review Example 7 (7.7) on =(-1)".Then on =O(1). lbn=1<M≡1.01 for all n≥1. Remark ●In the definition ofn=o(n入),the constant e can be set to be a very small value.Intuitively,bn=o(nA)implies that o grows at a rate strictly slower than n.That is, lim b n-oon =0. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 15/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 15/186 Example 7 (7.7) Limits and Orders of Magnitude: A Review Limits and Orders of Magnitude: A Review Remark