
CONTENTS 7.1 Limits and Orders of Magnitude:A Review 7.2 Motivation for Convergence Concepts 7.3 Convergence in Quadratic Mean and Lp-Convergence 7.4 Convergence in Probability 7.5 Almost Sure Convergence 7.6 Convergence in Distribution 7.7 Central Limit Theorems 7.8 Conclusion Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 21/186
Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 21/186 7.1 Limits and Orders of Magnitude: A Review 7.2 Motivation for Convergence Concepts 7.3 Convergence in Quadratic Mean and 𝑳𝑳𝒑𝒑-Convergence 7.4 Convergence in Probability 7.5 Almost Sure Convergence 7.6 Convergence in Distribution 7.7 Central Limit Theorems 7.8 Conclusion CONTENTS

Convergences and Limit Theorems Motivation for Convergence Concepts Motivation for Convergence Concepts Question:Why do we need convergence concepts in econo- metrics and statistics? ·A random sample Xm=(Xi,··,Xn)of size n is a se- quence of random variables X1,...,Xn.It can be viewed as an n-dimensional real-valued random vector,where the dimension n may go to infinity. Its realization is an n-dimensional vector x"=(1,...,n). A realization x"of Xm is usually called a sample point or a data set generated from the random sample X". Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 22/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 22/186 Motivation for Convergence Concepts Motivation for Convergence Concepts
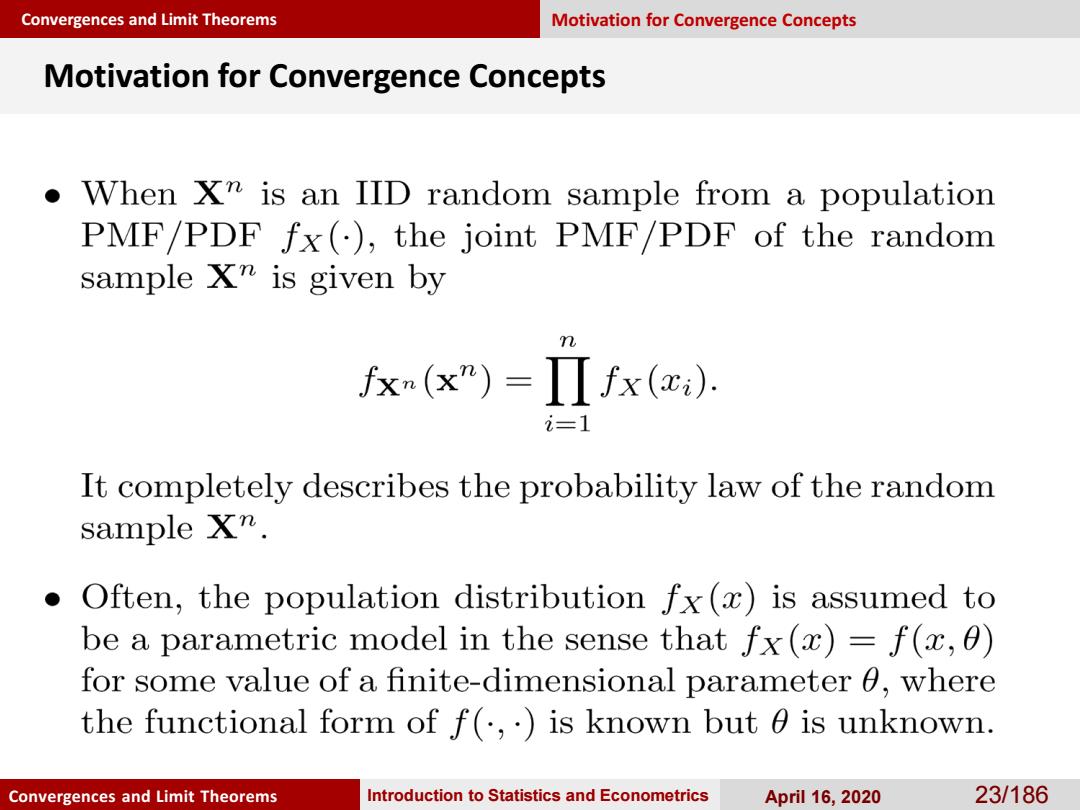
Convergences and Limit Theorems Motivation for Convergence Concepts Motivation for Convergence Concepts When X"is an IID random s sample from a population PMF/PDF fx(),the joint PMF/PDF of the random sample Xm is given by fxr(x")=fx(xi). 2=1 It completely describes the probability law of the random sample Xr. Often,the population distribution fx(x)is assumed to be a parametric model in the sense that fx(x)=f(x,0) for some value of a finite-dimensional parameter 0,where the functional form of f(,)is known but 0 is unknown. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 23/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 23/186 Motivation for Convergence Concepts Motivation for Convergence Concepts

Convergences and Limit Theorems Motivation for Convergence Concepts Motivation for Convergence Concepts For example,if fx(x)is assumed to follow a N(u,o2) distribution,we have fx(x)=f(x,0) c-(-), 1 1 V22 where 0 (u,o2). One important objective of statistical analysis is to es- timate the unknown parameter 0 when we are given a data set x",which is a realization of the random sample X".An estimator for 0 is a function of X,and so it is a statistic. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 24/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 24/186 Motivation for Convergence Concepts Motivation for Convergence Concepts

Convergences and Limit Theorems Motivation for Convergence Concepts Motivation for Convergence Concepts To motivate the importance of various convergence concepts,we con- sider two simple statistics-the sample mean and sample variance. They are used to estimate population mean u and population vari- ance o2 respectively. -The sample mean estimator Xn=T(X")=n1∑X: -1 The sample variance estimator S2=(m-1)-1(x-Xn)2. If the sample size n is sufficiently large,then it is expected that Xn will be“close”?to u and S2 will be“close”too2. Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16,2020 25/186
Convergences and Limit Theorems Convergences and Limit Theorems Introduction to Statistics and Econometrics April 16, 2020 25/186 Motivation for Convergence Concepts Motivation for Convergence Concepts