
OVERCONFIDENCE 1193 where wa is a standard Wiener process,and it is independent of in- novations to V.Trading Fluctuations in the difference of beliefs across agents will induce trading. It is natural to expect that investors that are more optimistic about the prospects of future dividends will bid up the price of the asset and eventually hold the total (finite)supply.We shall allow for costs of trading:a seller pays c20 per unit of the asset sold.This cost may represent an actual cost of transaction or a tax. At each agents in group Ce(A,B are willing to pay for a unit of the asset.The presence of the short-sale constraint,a finite supply of the asset,and an infinite number of prospective buyers guarantee that any successful bidder will pay his reservation price.The amount that an agent is willing to pay reflects the agent's fundamental valuation and the fact that he may be able to sell his holdings for a profit at a later date at the demand price of agents in the other group.If we let oE(A,B)denote the group of the current owner,o be the other group, and E be the expectation of members of group o,conditional on the information they have at t,then 书”=sup edD,+er(+,-) (10) 20 where r is a stopping time,and is the reservation value of the buyer at the time of transaction t+7. Since dD fdt+opdw,using the equations for the evolution of the conditional mean of beliefs (eqq.[5]and [9]above),we have that w= ee-Dlds+M where EM+,=0.Hence,we may rewrite equation (10)as p,"=max】 e-了+e9-d+e"(折,-0 (11) 20 We shall start by postulating a particular form for the equilibrium This observation simplifies our calculations but is not crucial for what follows.We could partially relax the short-sale constraints or the division of gains from trade,provided that it is still true that the asset owner expects to make speculative profits from other investors. Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission
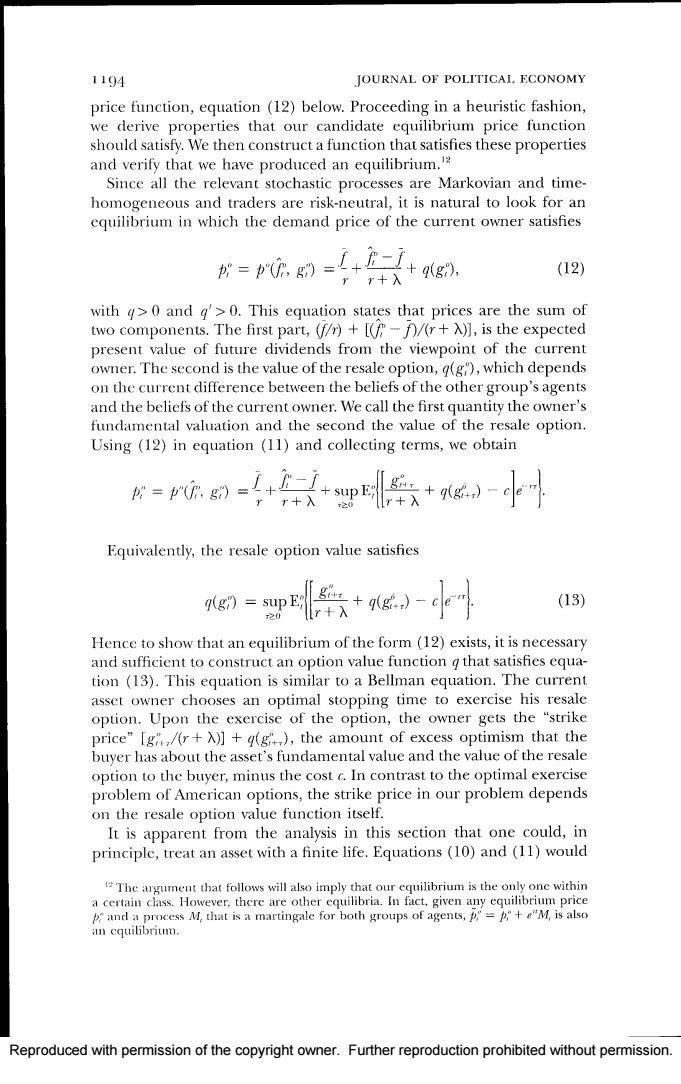
1194 JOURNAL OF POLITICAL.ECONOMY price function,equation (12)below.Proceeding in a heuristic fashion, we derive properties that our candidate equilibrium price function should satisfy.We then construct a function that satisfies these properties and verify that we have produced an equilibrium.2 Since all the relevant stochastic processes are Markovian and time- homogeneous and traders are risk-neutral,it is natural to look for an equilibrium in which the demand price of the current owner satisfies p g)g). (12) rr+入 with 4>0 and g'>0.This equation states that prices are the sum of two components.The first part,(fr)+[(-A)/(r+A)],is the expected present value of future dividends from the viewpoint of the current owner.The second is the value of the resale option,q(g"),which depends on the current difference between the beliefs of the other group's agents and the beliefs of the current owner.We call the first quantity the owner's fundamental valuation and the second the value of the resale option. Using (12)in equation (11)and collecting terms,we obtain Equivalently,the resale option value satisfies q(g= sup (13) Hence to show that an equilibrium of the form (12)exists,it is necessary and sufficient to construct an option value function g that satisfies equa- tion (13).This equation is similar to a Bellman equation.The current asset owner chooses an optimal stopping time to exercise his resale option.Upon the exercise of the option,the owner gets the "strike price”"[g,/r+入】+gg+,),the amount of excess optimism that the buyer has about the asset's fundamental value and the value of the resale option to the buyer,minus the cost c.In contrast to the optimal exercise problem of American options,the strike price in our problem depends on the resale option value function itself. It is apparent from the analysis in this section that one could,in principlc,treat an asset with a finite life.Equations(10)and (11)would The argument that follows will also imply that our cquilibrium is the only one within a certain class.However,there are other equilibria.In fact,given any equilibrium price p and a process M,that is a martingale for both groups of agents,p"=p+e"M,is also an cquilibrium. Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission
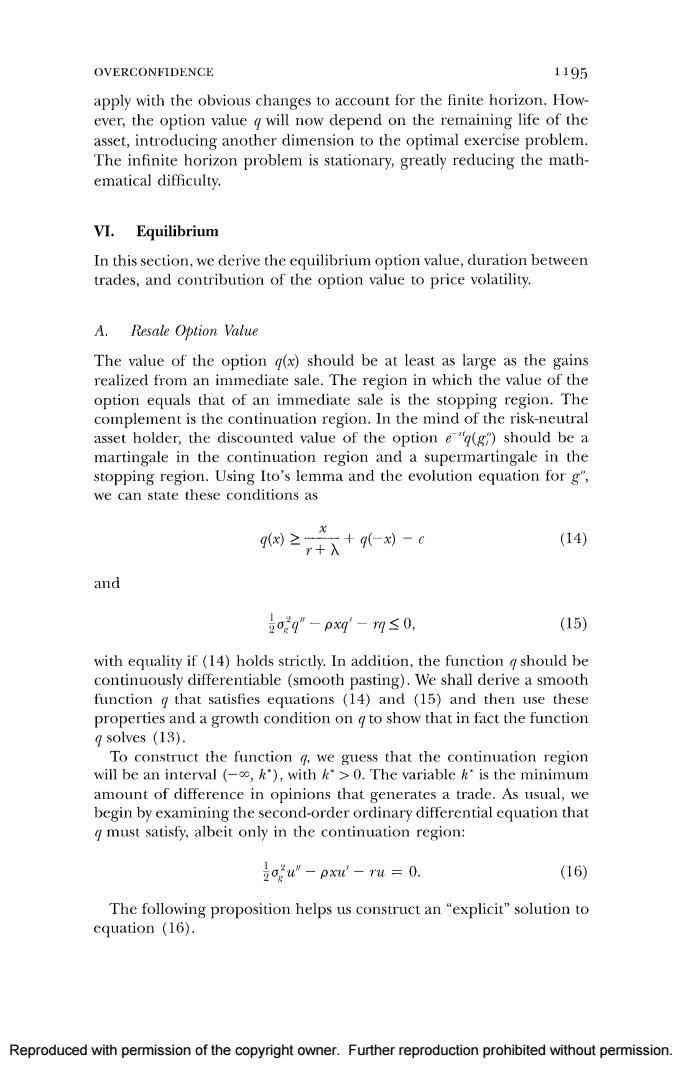
OVERCONFIDENCE 1195 apply with the obvious changes to account for the finite horizon.How- ever,the option value g will now depend on the remaining life of the asset,introducing another dimension to the optimal exercise problem. The infinite horizon problem is stationary,greatly reducing the math- ematical difficulty. VI.Equilibrium In this section,we derive the equilibrium option value,duration between trades,and contribution of the option value to price volatility. A. Resale Option Value The value of the option q(x)should be at least as large as the gains realized from an immediate sale.The region in which the value of the option equals that of an immediate sale is the stopping region.The complement is the continuation region.In the mind of the risk-neutral asset holder,the discounted value of the option e"g(g")should be a martingale in the continuation region and a supermartingale in the stopping region.Using Ito's lemma and the evolution equation for g", we can state these conditions as 9≥,+q9-c (14) r+λ and 豆0q”-pxq-q≤0, (15) with equality if(14)holds strictly.In addition,the function g should be continuously differentiable (smooth pasting).We shall derive a smooth function g that satisfies equations (14)and (15)and then use these properties and a growth condition on g to show that in fact the function g solves (13). To construct the function g,we guess that the continuation region will be an interval (-k'),with k'>0.The variable k'is the minimum amount of difference in opinions that generates a trade.As usual,we begin by examining the second-order ordinary differential equation that g must satisfy,albeit only in the continuation region: 2u"-pxu'-ru 0. (16) The following proposition helps us construct an"explicit"solution to cquation (16). Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission