
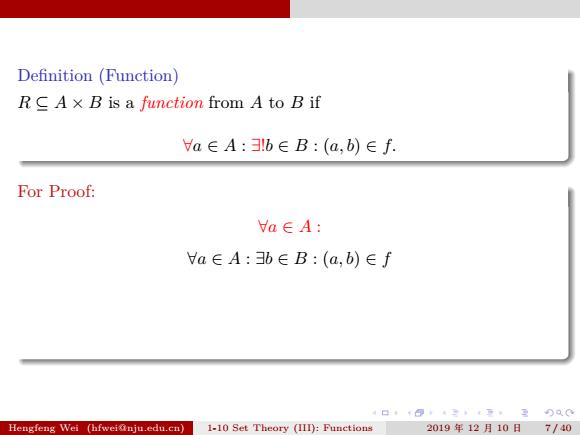
Definition (Function) RC A x B is a function from A to B if a∈A:b∈B:(a,b)∈f. For Proof: 4口·10,43,t夏,里Q0 Hengfeng Wei (hfweinju.edu.cn)1-10 Set Theory (III):Functions 2019年12月10日7/40
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Function) R ⊆ A × B is a function from A to B if ∀a ∈ A : ∃!b ∈ B : (a, b) ∈ f. For Proof: ∀a ∈ A : ∀a ∈ A : ∃b ∈ B : (a, b) ∈ f ∃!b ∈ B : ∀b, b ′ ∈ B : (a, b) ∈ f ∧ (a, b ′ ) ∈ f =⇒ b = b ′ Hengfeng Wei (hfwei@nju.edu.cn) 1-10 Set Theory (III): Functions 2019 年 12 月 10 日 7 / 40
Definition (Function) RC A x B is a function from A to B if a∈A:3b∈B:(a,b)∈f. For Proof: a∈A: a∈A:3b∈B:(a,b)∈f 4口·¥0,43,t夏,里Q0 Hengfeng Wei (hfweinju.edu.cn)1-10 Set Theory (III):Functions 2019年12月10日7/40
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Function) R ⊆ A × B is a function from A to B if ∀a ∈ A : ∃!b ∈ B : (a, b) ∈ f. For Proof: ∀a ∈ A : ∀a ∈ A : ∃b ∈ B : (a, b) ∈ f ∃!b ∈ B : ∀b, b ′ ∈ B : (a, b) ∈ f ∧ (a, b ′ ) ∈ f =⇒ b = b ′ Hengfeng Wei (hfwei@nju.edu.cn) 1-10 Set Theory (III): Functions 2019 年 12 月 10 日 7 / 40
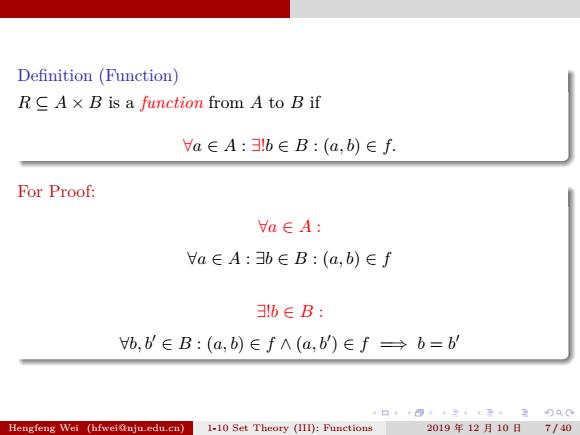
Definition (Function) RC A x B is a function from A to B if a∈A:3b∈B:(a,b)∈f. For Proof: Va∈A: a∈A:b∈B:(a,b)∈f 3b∈B: b,b∈B:(a,b)∈f∧(a,b)∈f→b=b 4口·¥①,4子,t夏3)Q0 Hengfeng Wei (hfweinju.edu.cn)1-10 Set Theory (III):Functions 2019年12月10日7/40
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Function) R ⊆ A × B is a function from A to B if ∀a ∈ A : ∃!b ∈ B : (a, b) ∈ f. For Proof: ∀a ∈ A : ∀a ∈ A : ∃b ∈ B : (a, b) ∈ f ∃!b ∈ B : ∀b, b′ ∈ B : (a, b) ∈ f ∧ (a, b′ ) ∈ f =⇒ b = b ′ Hengfeng Wei (hfwei@nju.edu.cn) 1-10 Set Theory (III): Functions 2019 年 12 月 10 日 7 / 40

Definition The set of all functions from X to Y: Yx={fIf:X→Y} 4口¥0,43,t夏,里Q0 Hengeng Wei thkweionjn.edu.cn 1-10 Set Theory (III):Functions 2019 1210 8/40
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition The set of all functions from X to Y : Y X = {f | f : X → Y } Y X = {f ∈ P(X × Y ) | f : X → Y } X and Y are finite sets with x and y elements, respectively. |X| = x |Y | = y, |Y X| = y x Hengfeng Wei (hfwei@nju.edu.cn) 1-10 Set Theory (III): Functions 2019 年 12 月 10 日 8 / 40
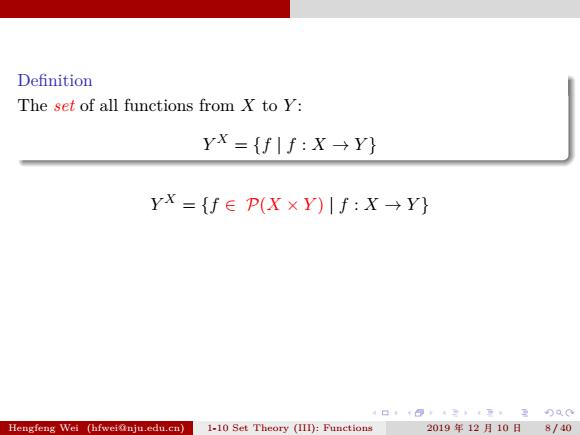
Definition The set of all functions from X to Y: Yx={f|f:X→Y} Yx={feP(X×Y)If:X→Y} 4口¥0,43,t夏,里Q0 Hengeng Wei thkweionjn.edu.cn 1-10 Set Theory (III):Functions 2019 1210 8/40
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition The set of all functions from X to Y : Y X = {f | f : X → Y } Y X = {f ∈ P(X × Y ) | f : X → Y } X and Y are finite sets with x and y elements, respectively. |X| = x |Y | = y, |Y X| = y x Hengfeng Wei (hfwei@nju.edu.cn) 1-10 Set Theory (III): Functions 2019 年 12 月 10 日 8 / 40