
CONTENTS CHAPTER 11 Properties of the Integers 264 11.1 Introduction 264 11.2 Order and Inequalities,Absolute Value 265 11.3 Mathematical Induction 266 11.4 Division Algorithm 267 11.5 Divisibility,Primes 269 11.6 Greatest Common Divisor,Euclidean Algorithm 270 11.7 Fundamental Theorem of Arithmetic 273 11.8 Congruence Relation 274 11.9 Congruence Equations 278 Solved Problems 283 Supplementary Problems 299 CHAPTER 12 Languages,Automata,Grammars 303 12.1 Introduction 303 12.2 Alphabet,Words,Free Semigroup 303 12.3 Languages 304 12.4 Regular Expressions,Regular Languages 305 12.5 Finite State Automata 306 12,6 Grammars 310 Solved Problems 314 Supplementary Problems 319 CHAPTER 13 Finite State Machines and Turing Machines 323 13.1 Introduction 323 13.2 Finite State Machines 323 13.3 Godel Numbers 326 13.4 Turing Machines 326 13.5 Computable Functions 330 Solved Problems 331 Supplementary Problems 334 CHAPTER 14 Ordered Sets and Lattices 337 14.1 Introduction 337 14.2 Ordered Sets 337 14.3 Hasse Diagrams of Partially Ordered Sets 340 14.4 Consistent Enumeration 342 14.5 Supremum and Infimum 342 14.6 Isomorphic (Similar)Ordered Sets 344 14.7 Well-Ordered Sets 344 14.8 Lattices 346 14.9 Bounded Lattices 348 14.10 Distributive Lattices 349 14.11 Complements,Complemented Lattices 350 Solved Problems Supplementary Problems 360
x CONTENTS CHAPTER 11 Properties of the Integers 264 11.1 Introduction 264 11.2 Order and Inequalities, Absolute Value 265 11.3 Mathematical Induction 266 11.4 Division Algorithm 267 11.5 Divisibility, Primes 269 11.6 Greatest Common Divisor, Euclidean Algorithm 270 11.7 Fundamental Theorem of Arithmetic 273 11.8 Congruence Relation 274 11.9 Congruence Equations 278 Solved Problems 283 Supplementary Problems 299 CHAPTER 12 Languages, Automata, Grammars 303 12.1 Introduction 303 12.2 Alphabet, Words, Free Semigroup 303 12.3 Languages 304 12.4 Regular Expressions, Regular Languages 305 12.5 Finite State Automata 306 12.6 Grammars 310 Solved Problems 314 Supplementary Problems 319 CHAPTER 13 Finite State Machines and Turing Machines 323 13.1 Introduction 323 13.2 Finite State Machines 323 13.3 Gödel Numbers 326 13.4 Turing Machines 326 13.5 Computable Functions 330 Solved Problems 331 Supplementary Problems 334 CHAPTER 14 Ordered Sets and Lattices 337 14.1 Introduction 337 14.2 Ordered Sets 337 14.3 Hasse Diagrams of Partially Ordered Sets 340 14.4 Consistent Enumeration 342 14.5 Supremum and Infimum 342 14.6 Isomorphic (Similar) Ordered Sets 344 14.7 Well-Ordered Sets 344 14.8 Lattices 346 14.9 Bounded Lattices 348 14.10 Distributive Lattices 349 14.11 Complements, Complemented Lattices 350 Solved Problems 351 Supplementary Problems 360

CONTENTS xi CHAPTER 15 Boolean Algebra 368 15.1 Introduction 368 15.2 Basic Definitions 368 15.3 Duality 369 15.4 Basic Theorems 370 15.5 Boolean Algebras as Lattices 370 15.6 Representation Theorem 371 15.7 Sum-of-Products Form for Sets 371 15.8 Sum-of-Products Form for Boolean Algebras 372 15.9 Minimal Boolean Expressions,Prime Implicants 375 15.10 Logic Gates and Circuits 377 15.11 Truth Tables,Boolean Functions 381 15.12 Karnaugh Maps 383 Solved Problems 389 Supplementary Problems 403 APPENDIX A Vectors and Matrices 409 A.1 Introduction 409 A.2 Vectors 409 A.3 Matrices 410 A.4 Matrix Addition and Scalar Multiplication 411 A.5 Matrix Multiplication 412 A.6 Transpose 414 A.7 Square Matrices 414 A.8 Invertible (Nonsingular)Matrices,Inverses 415 A.9 Determinants 416 A.10 Elementary Row Operations,Gaussian Elimination (Optional) 418 A.11 Boolean (Zero-One)Matrices 422 Solved Problems 423 Supplementary Problems 429 APPENDIX B Algebraic Systems 432 B.1 Introduction 432 B.2 Operations 432 B.3 Semigroups 435 B.4 Groups 438 B.5 Subgroups,Normal Subgroups,and Homomorphisms 440 B.6 Rings,Internal Domains,and Fields 443 B.7 Polynomials Over a Field 446 Solved Problems 450 Supplementary Problems 461 Index 467
CONTENTS xi CHAPTER 15 Boolean Algebra 368 15.1 Introduction 368 15.2 Basic Definitions 368 15.3 Duality 369 15.4 Basic Theorems 370 15.5 Boolean Algebras as Lattices 370 15.6 Representation Theorem 371 15.7 Sum-of-Products Form for Sets 371 15.8 Sum-of-Products Form for Boolean Algebras 372 15.9 Minimal Boolean Expressions, Prime Implicants 375 15.10 Logic Gates and Circuits 377 15.11 Truth Tables, Boolean Functions 381 15.12 Karnaugh Maps 383 Solved Problems 389 Supplementary Problems 403 APPENDIX A Vectors and Matrices 409 A.1 Introduction 409 A.2 Vectors 409 A.3 Matrices 410 A.4 Matrix Addition and Scalar Multiplication 411 A.5 Matrix Multiplication 412 A.6 Transpose 414 A.7 Square Matrices 414 A.8 Invertible (Nonsingular) Matrices, Inverses 415 A.9 Determinants 416 A.10 Elementary Row Operations, Gaussian Elimination (Optional) 418 A.11 Boolean (Zero-One) Matrices 422 Solved Problems 423 Supplementary Problems 429 APPENDIX B Algebraic Systems 432 B.1 Introduction 432 B.2 Operations 432 B.3 Semigroups 435 B.4 Groups 438 B.5 Subgroups, Normal Subgroups, and Homomorphisms 440 B.6 Rings, Internal Domains, and Fields 443 B.7 Polynomials Over a Field 446 Solved Problems 450 Supplementary Problems 461 Index 467

CHAPTER 1 Set Theory 1.1 INTRODUCTION The concept of a set appears in all mathematics.This chapter introduces the notation and terminology of set theory which is basic and used throughout the text.The chapter closes with the formal definition of mathematical induction,with examples 1.2 SETS AND ELEMENTS.SUBSETS A set may be viewed as any well-defined collection of objects,called the elements or members of the set. One usually uses capital letters,A.B.X.Y.....to denote sets,and lowercase letters,a,b,x,y....,to denote elements of sets.Synonyms for“set”are“class,”“collection,.”and“family." Membership in a set is denoted as follows: a e S denotes that a belongs to a set S a,bS denotes that a and b belong to a set S Here∈is the symbol meaning“is an element of..”We use年to mean“is not an element of..” Specifying Sets There are essentially two ways to specify a particular set.One way,if possible,is to list its members separated by commas and contained in braces {)Asecond way is to state those properties which characterized the elements in the set.Examples illustrating these two ways are: A=(1,3,5,7,9)and B={x Ix is an even integer,x>0) That is.A consists of the numbers 1.3.5.7.9.The second set.which reads: B is the set of x such that x is an even integer and x is greater than 0, denotes the set B whose elements are the positive integers.Note that a letter,usuallyx,is used to denote a typical member of the set;and the vertical line|is read as“such that'”and the comma as“and.” EXAMPLE 1.1 (a)The set A above can also be written as A={xIx is an odd positive integer,x 10). (b)We cannot list all the elements of the above set B although frequently we specify the set by B={2,4,6,} where we assume that everyone knows what we mean.Observe that 8 B,but 3B. Copyright@2007,1997,1976 by The McGraw-Hill Companies,Inc.Click here for terms of use
CHAPTER 1 Set Theory 1.1 INTRODUCTION The concept of a set appears in all mathematics. This chapter introduces the notation and terminology of set theory which is basic and used throughout the text. The chapter closes with the formal definition of mathematical induction, with examples. 1.2 SETS AND ELEMENTS, SUBSETS A set may be viewed as any well-defined collection of objects, called the elements or members of the set. One usually uses capital letters, A, B, X, Y, . . . , to denote sets, and lowercase letters, a, b, x, y, . . ., to denote elements of sets. Synonyms for “set” are “class,” “collection,” and “family.” Membership in a set is denoted as follows: a ∈ S denotes that a belongs to a set S a, b ∈ S denotes that a and b belong to a set S Here ∈ is the symbol meaning “is an element of.” We use ∈ to mean “is not an element of.” Specifying Sets There are essentially two ways to specify a particular set. One way, if possible, is to list its members separated by commas and contained in braces { }. A second way is to state those properties which characterized the elements in the set. Examples illustrating these two ways are: A = {1, 3, 5, 7, 9} and B = {x | x is an even integer, x > 0} That is, A consists of the numbers 1, 3, 5, 7, 9. The second set, which reads: B is the set of x such that x is an even integer and x is greater than 0, denotes the set B whose elements are the positive integers. Note that a letter, usually x, is used to denote a typical member of the set; and the vertical line | is read as “such that” and the comma as “and.” EXAMPLE 1.1 (a) The set A above can also be written as A = {x | x is an odd positive integer, x < 10}. (b) We cannot list all the elements of the above set B although frequently we specify the set by B = {2, 4, 6,...} where we assume that everyone knows what we mean. Observe that 8 ∈ B, but 3 ∈/ B. 1 Copyright © 2007, 1997, 1976 by The McGraw-Hill Companies, Inc. Click here for terms of use. �

2 SET THEORY [CHAP.1 (c)Let E ={xlx2-3x+2=0),F=(2,1)and G=(1,2,2,1).Then E=F=G We emphasize that a set does not depend on the way in which its elements are displayed.A set remains the same if its elements are repeated or rearranged. Even if we can list the elements of a set,it may not be practical to do so.That is,we describe a set by listing its elements only if the set contains a few elements;otherwise we describe a set by the property which characterizes its elements. Subsets Suppose every element in a set A is also an element of a set B,that is,suppose aA implies a B.Then A is called a subset of B.We also say that A is contained in B or that B contains A.This relationship is written A≤BorB2A Two sets are equal if they both have the same elements or,equivalently,if each is contained in the other.That is: A=B if and only if A≤B and B∈A If A is not a subset of B,that is,if at least one element of A does not belong to B,we write A g B. EXAMPLE 1.2 Consider the sets: A={1,3,4,7,8,91,B={1,2,3,4,5,C={1,3} Then CC A and CC B since 1 and 3,the elements of C,are also members of A and B.But B A since some of the elements of B,e.g.,2 and 5,do not belong to A.Similarly,A B. Property 1:It is common practice in mathematics to put a vertical line""or slanted line""through a symbol to indicate the opposite or negative meaning of a symbol. Property 2:The statement A B does not exclude the possibility that A=B.In fact,for every set A we have A C A since,trivially,every element in A belongs to A.However,if A C B and A B,then we say A is a proper subset of B(sometimes written AC B). Property 3:Suppose every element of a set A belongs to a set B and every element of B belongs to a set C. Then clearly every element of A also belongs to C.In other words,if A B and BCC,then A CC. The above remarks yield the following theorem. Theorem 1.1:Let A,B,C be any sets.Then: ①)A≤A (i)IfA≤B and B≤A,then A=B (ii)IfA≤B and B≤C,then A∈C Special symbols Some sets will occur very often in the text,and so we use special symbols for them.Some such symbols are: N=the set of natural numbers or positive integers:1,2,3.... Z=the set of all integers:...,-2,-1,0,1,2,... Q the set of rational numbers R=the set of real numbers C=the set of complex numbers Observe that NCZCQCRC C
2 SET THEORY [CHAP. 1 (c) Let E = {x | x2 − 3x + 2 = 0}, F = {2, 1} and G = {1, 2, 2, 1}. Then E = F = G. We emphasize that a set does not depend on the way in which its elements are displayed. A set remains the same if its elements are repeated or rearranged. Even if we can list the elements of a set, it may not be practical to do so. That is, we describe a set by listing its elements only if the set contains a few elements; otherwise we describe a set by the property which characterizes its elements. Subsets Suppose every element in a set A is also an element of a set B, that is, suppose a ∈ A implies a ∈ B. Then A is called a subset of B. We also say that A is contained in B or that B contains A. This relationship is written A ⊆ B or B ⊇ A Two sets are equal if they both have the same elements or, equivalently, if each is contained in the other. That is: A = B if and only if A ⊆ B and B ⊆ A If A is not a subset of B, that is, if at least one element of A does not belong to B, we write A ⊆ B. EXAMPLE 1.2 Consider the sets: A = {1, 3, 4, 7, 8, 9}, B = {1, 2, 3, 4, 5}, C = {1, 3}. Then C ⊆ A and C ⊆ B since 1 and 3, the elements of C, are also members of A and B. But B ⊆ A since some of the elements of B, e.g., 2 and 5, do not belong to A. Similarly, A ⊆ B. Property 1: It is common practice in mathematics to put a vertical line “|” or slanted line “/” through a symbol to indicate the opposite or negative meaning of a symbol. Property 2: The statement A ⊆ B does not exclude the possibility that A = B. In fact, for every set A we have A ⊆ A since, trivially, every element in A belongs to A. However, if A ⊆ B and A = B, then we say A is a proper subset of B (sometimes written A ⊂ B). Property 3: Suppose every element of a set A belongs to a set B and every element of B belongs to a set C. Then clearly every element of A also belongs to C. In other words, if A ⊆ B and B ⊆ C, then A ⊆ C. The above remarks yield the following theorem. Theorem 1.1: Let A, B, C be any sets. Then: (i) A ⊆ A (ii) If A ⊆ B and B ⊆ A, then A = B (iii) If A ⊆ B and B ⊆ C, then A ⊆ C Special symbols Some sets will occur very often in the text, and so we use special symbols for them. Some such symbols are: N = the set of natural numbers or positive integers: 1, 2, 3,... Z = the set of all integers: ..., −2, −1, 0, 1, 2,... Q = the set of rational numbers R = the set of real numbers C = the set of complex numbers Observe that N ⊆ Z ⊆ Q ⊆ R ⊆ C.����
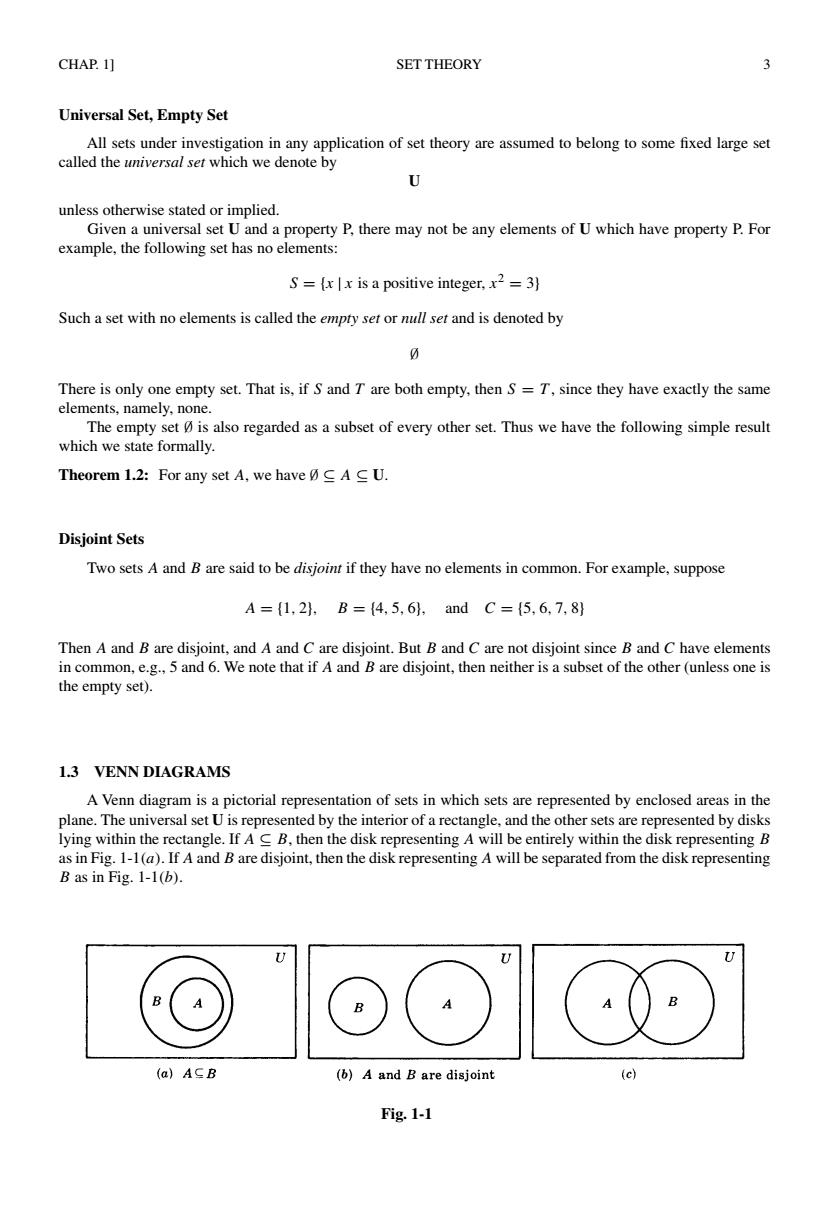
CHAP.1] SET THEORY 3 Universal Set,Empty Set All sets under investigation in any application of set theory are assumed to belong to some fixed large set called the universal set which we denote by ◇ unless otherwise stated or implied. Given a universal set U and a property P,there may not be any elements of U which have property P.For example,the following set has no elements: S=xx is a positive integer,x2=3} Such a set with no elements is called the empty set or null set and is denoted by 分 There is only one empty set.That is,if S and T are both empty,then S=T,since they have exactly the same elements,namely,none. The empty set is also regarded as a subset of every other set.Thus we have the following simple result which we state formally. Theorem 1.2:For any set A,we have 0A C U. Disjoint Sets Two sets A and B are said to be disjoint if they have no elements in common.For example,suppose A={1,2,B={4,5,6,andC={5,6,7,8} Then A and B are disjoint,and A and C are disjoint.But B and C are not disjoint since B and C have elements in common,e.g.,5 and 6.We note that if A and B are disjoint,then neither is a subset of the other (unless one is the empty set). 1.3 VENN DIAGRAMS A Venn diagram is a pictorial representation of sets in which sets are represented by enclosed areas in the plane.The universal set U is represented by the interior of a rectangle,and the other sets are represented by disks lying within the rectangle.If A C B,then the disk representing A will be entirely within the disk representing B as in Fig.1-1(a).If A and B are disjoint,then the disk representing A will be separated from the disk representing B as in Fig.1-1(b). B (a)ACB (b)A and B are disjoint (c) Fig.1-1
CHAP. 1] SET THEORY 3 Universal Set, Empty Set All sets under investigation in any application of set theory are assumed to belong to some fixed large set called the universal set which we denote by U unless otherwise stated or implied. Given a universal set U and a property P, there may not be any elements of U which have property P. For example, the following set has no elements: S = {x | x is a positive integer, x2 = 3} Such a set with no elements is called the empty set or null set and is denoted by ∅ There is only one empty set. That is, if S and T are both empty, then S = T , since they have exactly the same elements, namely, none. The empty set ∅ is also regarded as a subset of every other set. Thus we have the following simple result which we state formally. Theorem 1.2: For any set A, we have ∅ ⊆ A ⊆ U. Disjoint Sets Two sets A and B are said to be disjoint if they have no elements in common. For example, suppose A = {1, 2}, B = {4, 5, 6}, and C = {5, 6, 7, 8} Then A and B are disjoint, and A and C are disjoint. But B and C are not disjoint since B and C have elements in common, e.g., 5 and 6. We note that if A and B are disjoint, then neither is a subset of the other (unless one is the empty set). 1.3 VENN DIAGRAMS A Venn diagram is a pictorial representation of sets in which sets are represented by enclosed areas in the plane. The universal set U is represented by the interior of a rectangle, and the other sets are represented by disks lying within the rectangle. If A ⊆ B, then the disk representing A will be entirely within the disk representing B as in Fig. 1-1(a). If A and B are disjoint, then the disk representing A will be separated from the disk representing B as in Fig. 1-1(b). Fig. 1-1