
Outline Sets Relations Functions Products Sums 2/40
Outline Sets Relations Functions Products Sums 2 / 40

Sets -Basic Notations x∈S membership SCT subset SCT proper subset S Cfin T finite subset S=T equivalence 0 the empty set N natural numbers Z integers B [true,false 4/40
Sets – Basic Notations x ∈ S membership S ⊆ T subset S ⊂ T proper subset S ⊆fin T finite subset S = T equivalence ∅ the empty set N natural numbers Z integers B {true,false} 4 / 40

Sets -Basic Notations SnT intersection sr{xIx∈S and x∈T} SUT union s{x xES orx∈T} S-T difference 些{xIx∈S and x年T} P(S) powerset ef{TlT≤S} [m,n] integer range s{x|m≤x≤n 5/40
Sets – Basic Notations S ∩ T intersection def = {x | x ∈ S and x ∈ T} S ∪ T union def = {x | x ∈ S or x ∈ T} S − T difference def = {x | x ∈ S and x 6∈ T} P(S) powerset def = {T | T ⊆ S} [m, n] integer range def = {x | m ≤ x ≤ n} 5 / 40
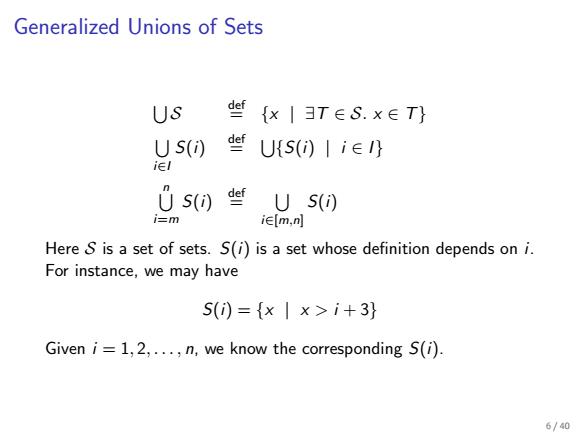
Generalized Unions of Sets US s{x|3T∈S.x∈T} U5(i) d UfS(i)Iiel iel 050些 U s(i) i=m ie[m,n Here S is a set of sets.S(i)is a set whose definition depends on i. For instance,we may have S(i)={x|x>i+3} Given i=1,2,...,n,we know the corresponding S(i). 6/40
Generalized Unions of Sets S S def = {x | ∃T ∈ S. x ∈ T} S i∈I S(i) def = S {S(i) | i ∈ I } Sn i=m S(i) def = S i∈[m,n] S(i) Here S is a set of sets. S(i) is a set whose definition depends on i. For instance, we may have S(i) = {x | x > i + 3} Given i = 1, 2, . . . , n, we know the corresponding S(i). 6 / 40

Generalized Unions of Sets Example(1) AUB=A,B} Proof? Example (2) LetS(i)=[i,i+1]andI={U2Ij∈[1,3]},then US(i)={1,2,4,5,9,10} iel 1/40
Generalized Unions of Sets Example (1) A ∪ B = [ {A, B} Proof? Example (2) Let S(i) = [i, i + 1] and I = {j 2 | j ∈ [1, 3]}, then [ i∈I S(i) = {1, 2, 4, 5, 9, 10} 7 / 40