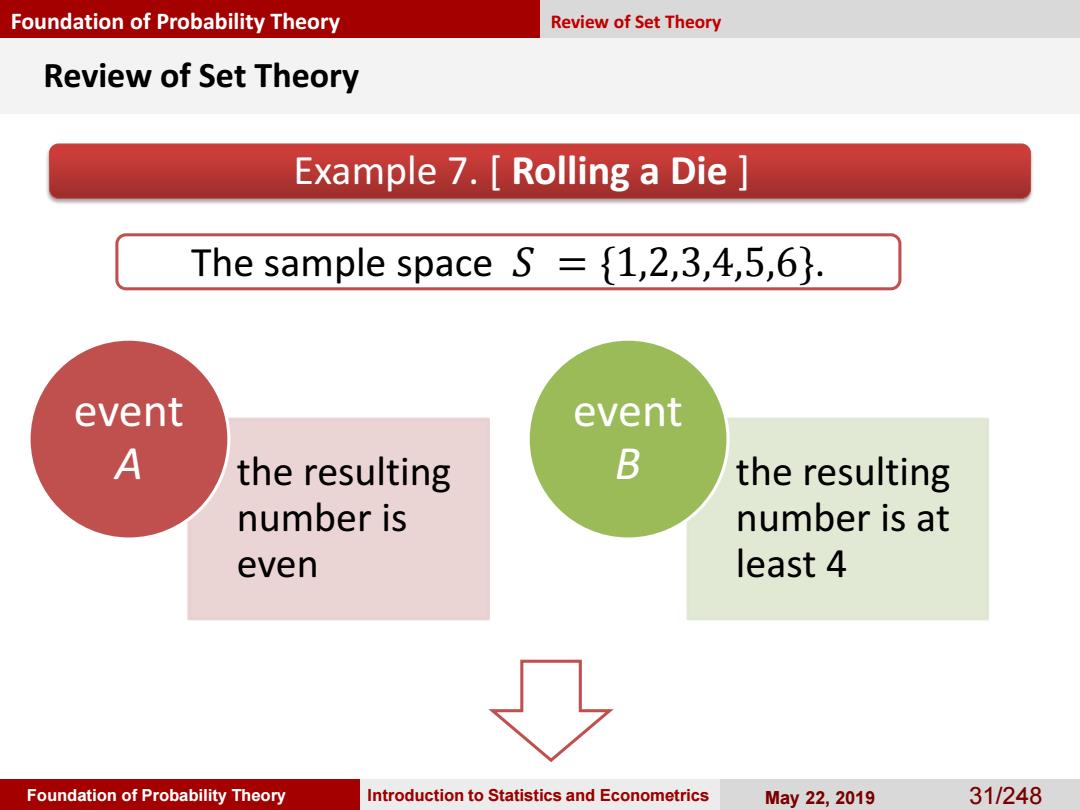
Foundation of Probability Theory Review of Set Theory Review of Set Theory Example 7.Rolling a Die The sample space S ={1,2,3,4,5,61. event event A the resulting the resulting number is number is at even least 4 Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 31/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 31/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Example 7. [ Rolling a Die ] The sample space 𝑆 = {1,2,3,4,5,6}. the resulting number is even event A the resulting number is at least 4 event B

Foundation of Probability Theory Review of Set Theory Review of Set Theory Then it follows that A={2,4,6}, B={4,5,6, Ac={1,3,5, B={1,2,3, A-B={2, B-A={5}, A∩B={4,6, AUB={2,4,5,6}, A∩Ac=0, AUAc={1,2,3,4,5,6}=S. Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 32/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 32/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Example 8. [ Rolling a Die ] 𝐴 = 2,4,6 , 𝐴 𝑐 = 1,3,5 , 𝐴 − 𝐵 = 2 , 𝐴 ∩ 𝐵 = 4,6 , 𝐴 ∩ 𝐴 𝑐 = ∅ , 𝐵 = 4,5,6 , 𝐵 = 1,2,3 , 𝐵 − 𝐴 = 5 , 𝐴 ∪ 𝐵 = 2,4,5,6 , 𝐴 ∪ 𝐴 𝑐 = 1,2,3,4,5,6 = 𝑆. Then it follows that

Foundation of Probability Theory Review of Set Theory Review of Set Theory Theorem 1.Laws of Sets Operations For any three events A,B,C defined on a sample space S; Complementation -A 0c S 三 0 Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 33/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 33/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Theorem 1. [ Laws of Sets Operations ] For any three events A, B, C defined on a sample space S; Complementation 𝐴 𝑐 𝑐 = 𝐴 ∅ 𝑐 = 𝑆 𝑆 𝑐 = ∅
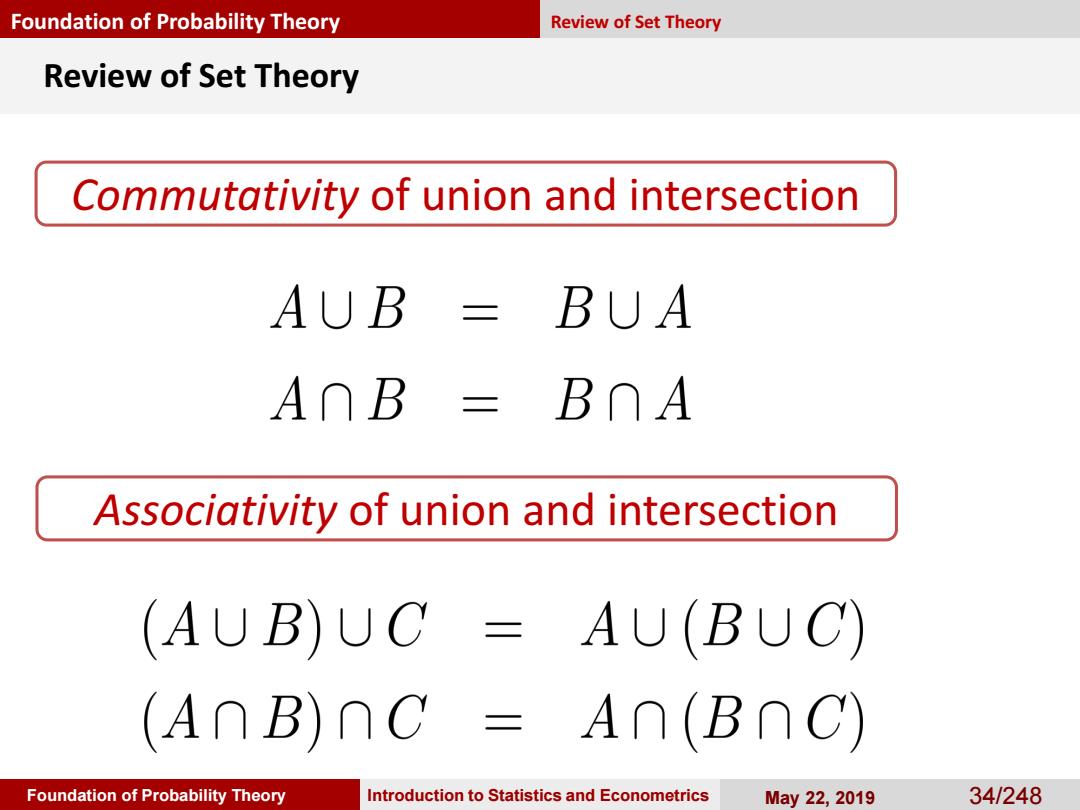
Foundation of Probability Theory Review of Set Theory Review of Set Theory Commutativity of union and intersection AUB BUA A∩B 三 B∩A Associativity of union and intersection (AUB)UC AU(BUC) (A∩B)∩C =A∩(B∩C) Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 34/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 34/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Commutativity of union and intersection Associativity of union and intersection
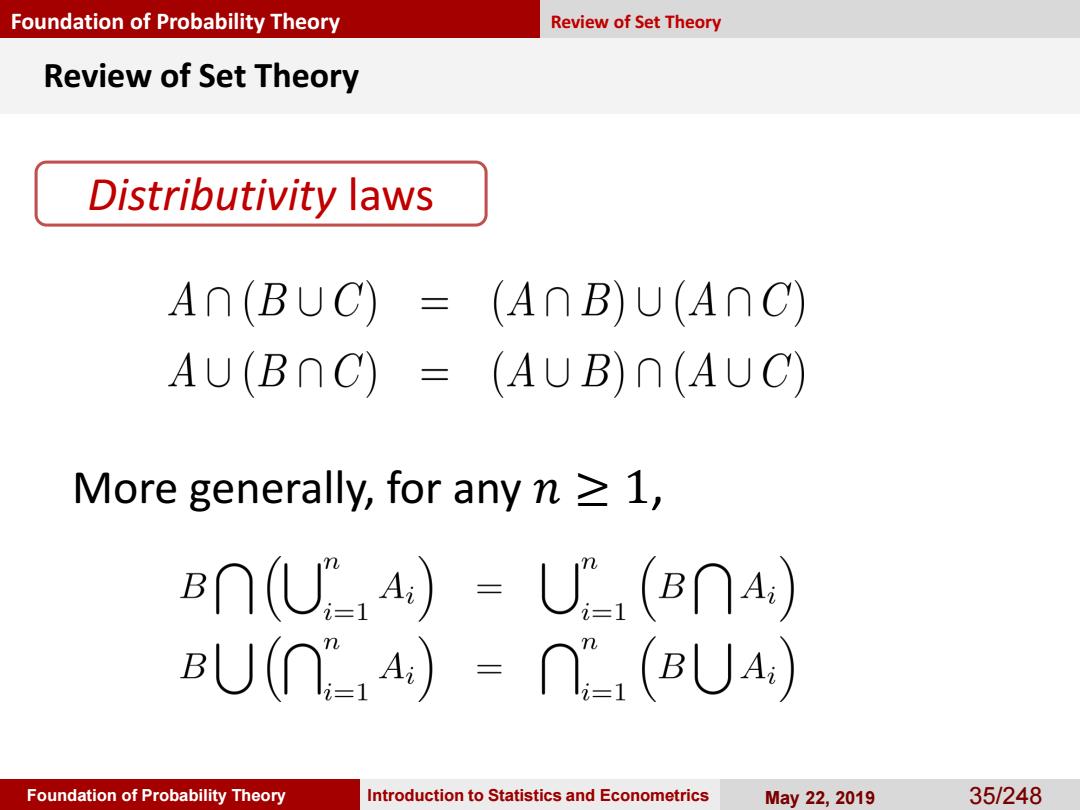
Foundation of Probability Theory Review of Set Theory Review of Set Theory Distributivity laws A∩(BUC)= (A∩B)U(A∩C) AU(BnC)=(AUB)n(AUC) More generally,for any n >1, B∩U,A)=U,(B∩A,〉 BU(∩”,A)=∩”,(BUA:) Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 35/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 35/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Distributivity laws More generally, for any 𝑛 ≥ 1