
CONTENTS 2.1 Random Experiments 2.2 Basic Concepts of Probability 2.3 Review of Set Theory 2.4 Fundamental Probability Laws 2.5 Methods of Counting 2.6 Conditional Probability 2.7 Bayes'Theorem 2.8 Independence 2.9 Conclusion Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 21/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 21/248 2.1 Random Experiments 2.2 Basic Concepts of Probability 2.3 Review of Set Theory 2.4 Fundamental Probability Laws 2.5 Methods of Counting 2.6 Conditional Probability 2.7 Bayes' Theorem 2.8 Independence 2.9 Conclusion CONTENTS
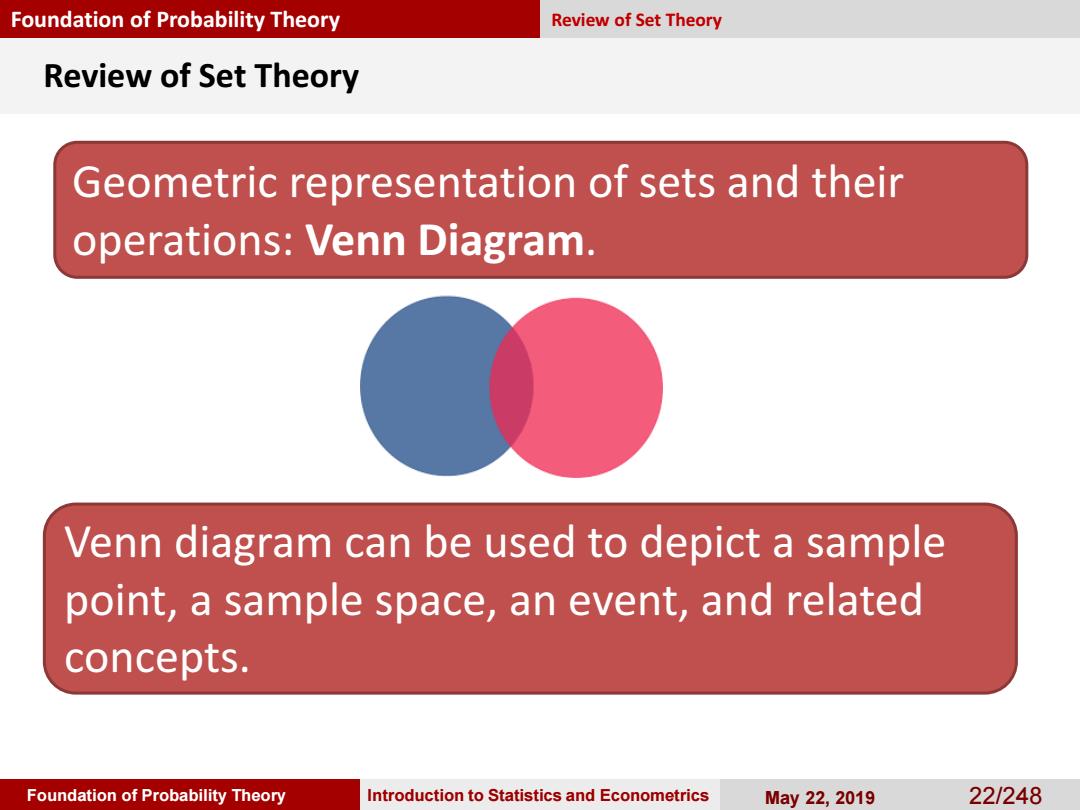
Foundation of Probability Theory Review of Set Theory Review of Set Theory Geometric representation of sets and their operations:Venn Diagram. Venn diagram can be used to depict a sample point,a sample space,an event,and related concepts. Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 22/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 22/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Geometric representation of sets and their operations: Venn Diagram. Venn diagram can be used to depict a sample point, a sample space, an event, and related concepts
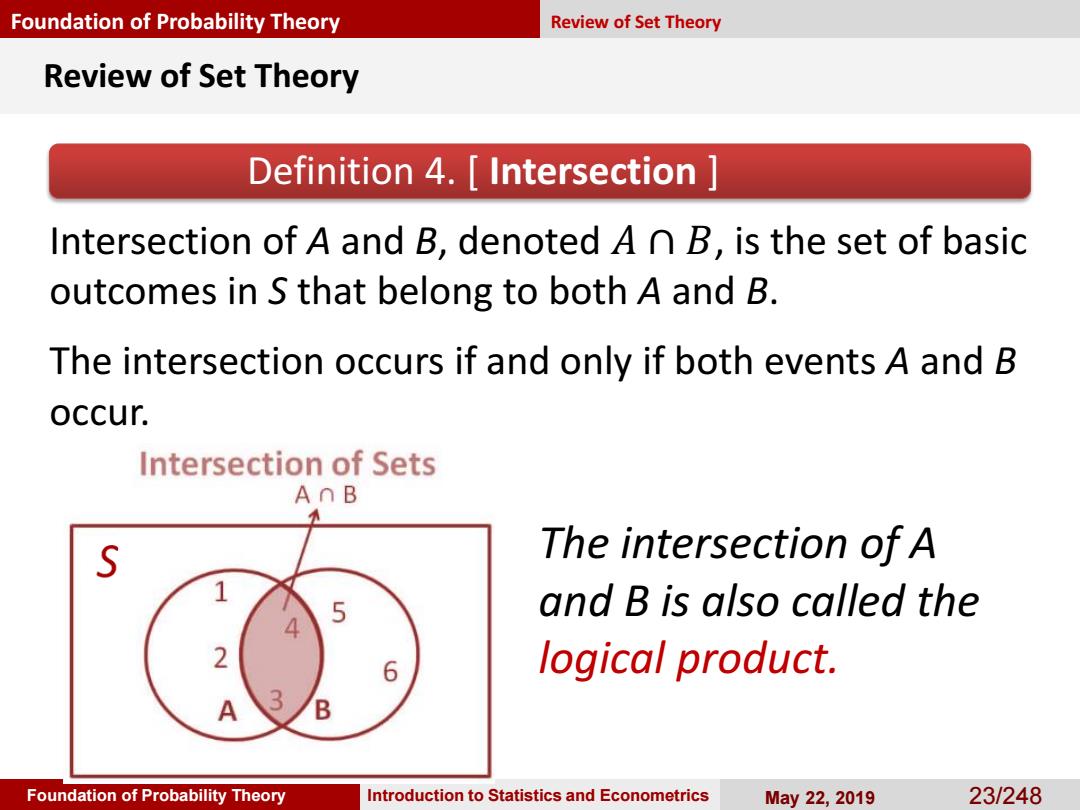
Foundation of Probability Theory Review of Set Theory Review of Set Theory Definition 4.Intersection Intersection of A and B,denoted A n B,is the set of basic outcomes in S that belong to both A and B. The intersection occurs if and only if both events A and B occur. Intersection of Sets AnB S The intersection of A and B is also called the 6 logical product. B Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 23/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 23/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Definition 4. [ Intersection ] Intersection of A and B, denoted 𝐴 ∩ 𝐵, is the set of basic outcomes in S that belong to both A and B. The intersection occurs if and only if both events A and B occur. The intersection of A and B is also called the logical product

Foundation of Probability Theory Review of Set Theory Review of Set Theory Definition 5.Exclusiveness If A and B have no common basic outcomes,they are called mutually exclusive and their intersection is empty set 0,i.e.,An B =0,where 0 denotes an empty set that contains nothing. S 2 3 A B Disjoint Sets Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 24/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 24/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Definition 5. [ Exclusiveness ] If A and B have no common basic outcomes, they are called mutually exclusive and their intersection is empty set ∅, i.e., 𝐴 ∩ B = ∅, where ∅ denotes an empty set that contains nothing

Foundation of Probability Theory Review of Set Theory Review of Set Theory Remarks: Mutually exclusive events are also called disjoint because they do not overlap when represented in the Venn diagram. Any mutually exclusive events cannot occur simultaneously.As an example,any pair of the basic outcomes in sample space S are mutually exclusive. Foundation of Probability Theory Introduction to Statistics and Econometrics May22,2019 25/248
Foundation of Probability Theory Introduction to Statistics and Econometrics May 22, 2019 25/248 Review of Set Theory Foundation of Probability Theory Review of Set Theory Remarks: ● Mutually exclusive events are also called disjoint because they do not overlap when represented in the Venn diagram. ● Any mutually exclusive events cannot occur simultaneously. As an example, any pair of the basic outcomes in sample space S are mutually exclusive