
Bounding the Distance between a Loop Subdivision Surface and Its Limit Mesh Zhangjin Huang Guoping Wang HCI Multimedia Lab Peking University,P.R.China
Bounding the Distance between a Loop Subdivision Surface and Its Limit Mesh Zhangjin Huang Guoping Wang HCI & Multimedia Lab Peking University, P.R. China
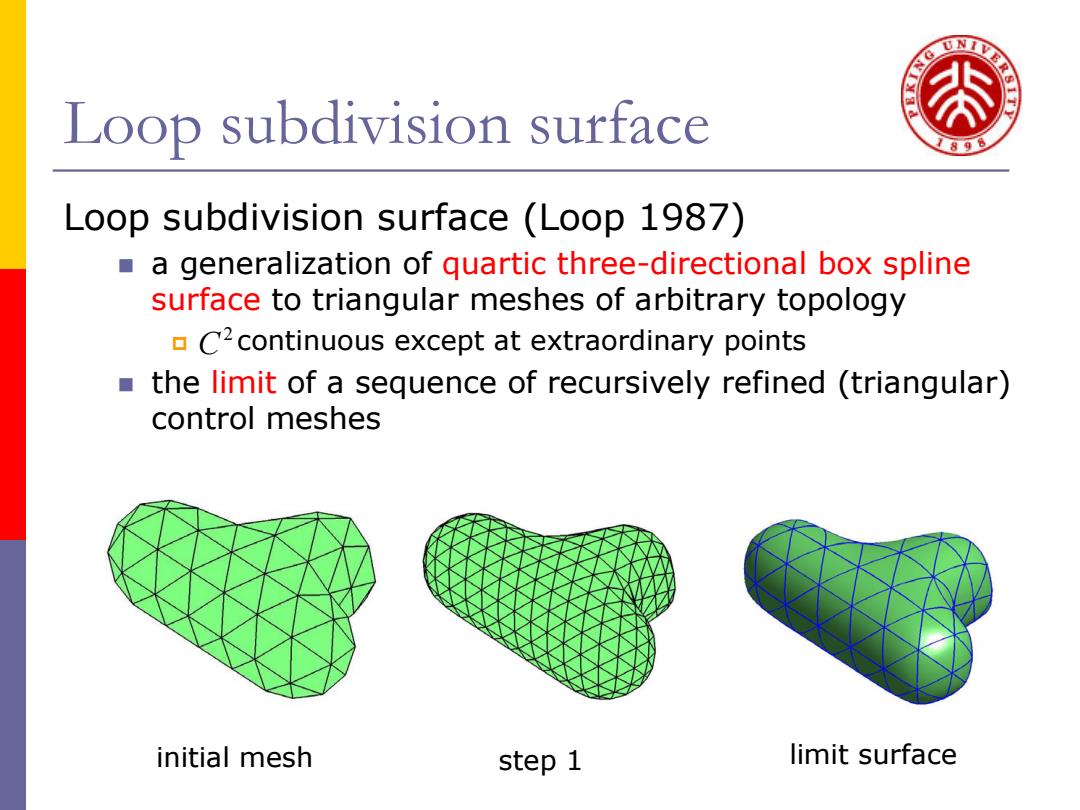
Loop subdivision surface 89 Loop subdivision surface (Loop 1987) a generalization of quartic three-directional box spline surface to triangular meshes of arbitrary topology C2continuous except at extraordinary points the limit of a sequence of recursively refined (triangular) control meshes initial mesh step 1 limit surface
Loop subdivision surface Loop subdivision surface (Loop 1987) a generalization of quartic three-directional box spline surface to triangular meshes of arbitrary topology continuous except at extraordinary points the limit of a sequence of recursively refined (triangular) control meshes 2 C initial mesh step 1 limit surface
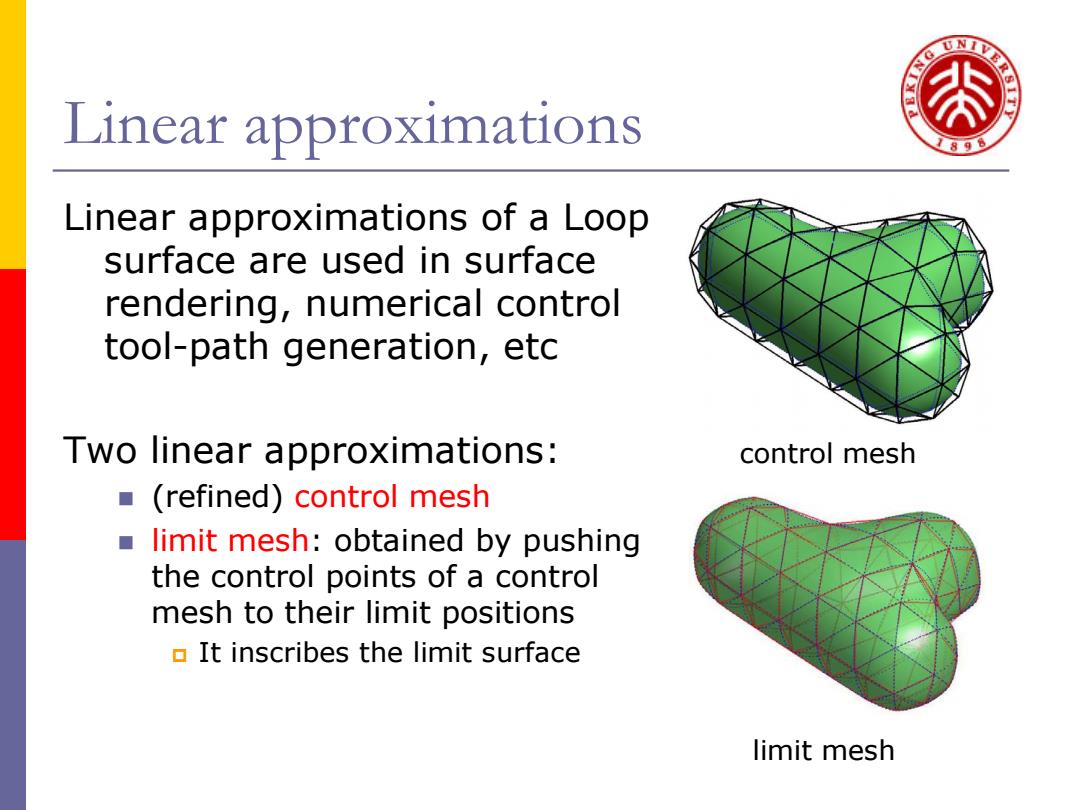
U N I Linear approximations 89了 Linear approximations of a Loop surface are used in surface rendering,numerical control tool-path generation,etc Two linear approximations: control mesh (refined)control mesh limit mesh:obtained by pushing the control points of a control mesh to their limit positions It inscribes the limit surface limit mesh
Linear approximations Linear approximations of a Loop surface are used in surface rendering, numerical control tool-path generation, etc Two linear approximations: (refined) control mesh limit mesh: obtained by pushing the control points of a control mesh to their limit positions It inscribes the limit surface control mesh limit mesh
Problems of limit mesh approx. Approximation error How to estimate the error(distance) between a Loop surface and its limit mesh? Subdivision depth estimation How many steps of subdivision would be necessary to satisfy a user-specified error tolerance?
Problems of limit mesh approx. Approximation error How to estimate the error (distance) between a Loop surface and its limit mesh? Subdivision depth estimation How many steps of subdivision would be necessary to satisfy a user-specified error tolerance?
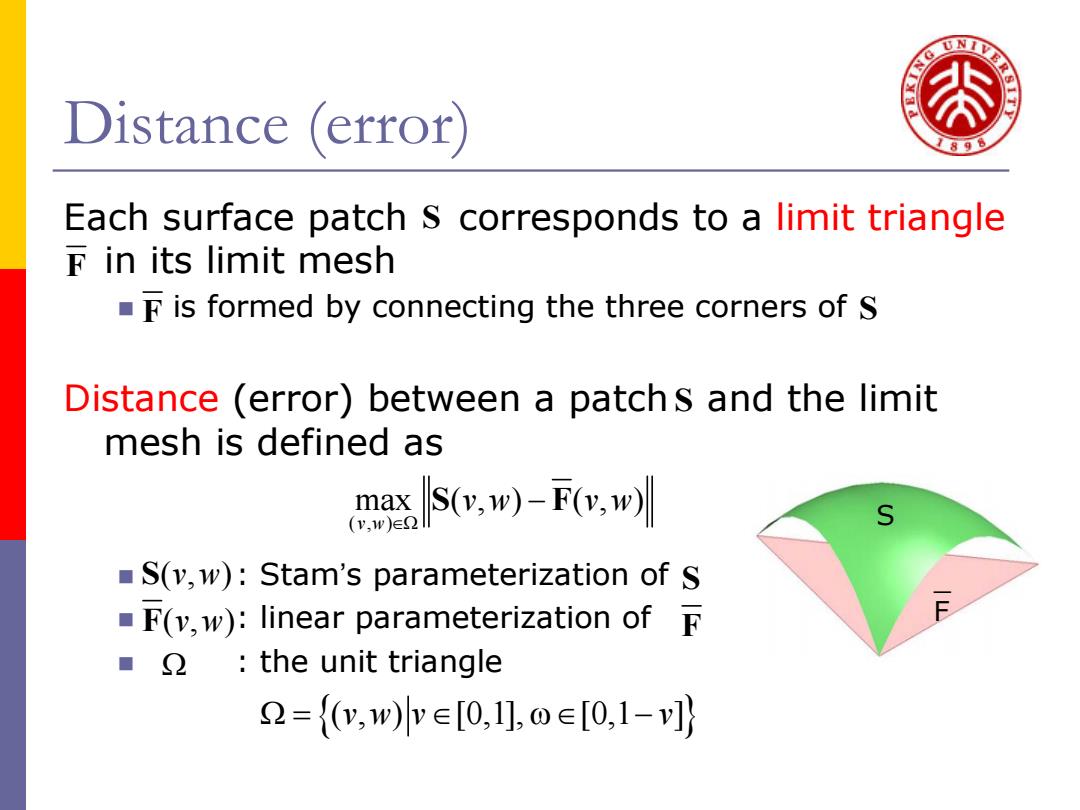
Distance (error) 89 Each surface patch S corresponds to a limit triangle F in its limit mesh F is formed by connecting the three corners of S Distance (error)between a patchs and the limit mesh is defined as maS0)-F,w S(v,w):Stam's parameterization of S F(v,w):linear parameterization ofF ■2:the unit triangle 2={(y,w)p∈[0,1,o∈[0,1-
Distance (error) Each surface patch corresponds to a limit triangle in its limit mesh is formed by connecting the three corners of Distance (error) between a patch and the limit mesh is defined as : Stam’s parameterization of : linear parameterization of : the unit triangle S(, ) v w Ω Ω = ∈ ω∈ − {( , ) [0,1], [0,1 ] vw v v } F(, ) v w S F (, ) max ( , ) ( , ) v w vw vw ∈Ω S F− S F F S S S _ F