Definition (Range) ran(R)={ba (a,b)ER} Theorem ran(R)is a set. ran(R)={b∈U儿UR|3a:(a,b)∈R Definition (Field) fid(R)=dom(R)Uran(R) Hengfeng Wei (hfweixinju.edu.cn) 1-9 Set Theory (II):Relations 2019年12月03日16/51
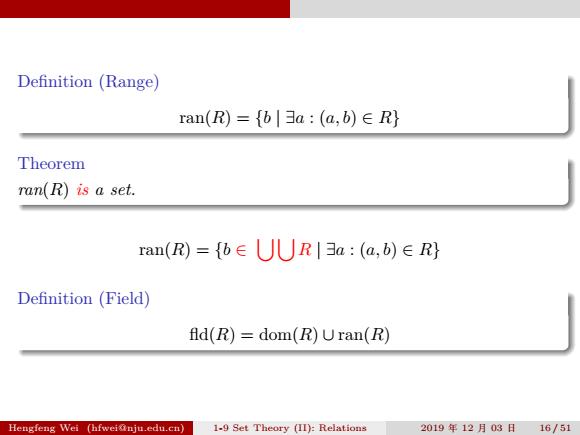
Definition (Range) ran(R) = {b | ∃a : (a, b) ∈ R} Theorem ran(R) is a set. ran(R) = {b ∈ ∪∪R | ∃a : (a, b) ∈ R} Definition (Field) fld(R) = dom(R) ∪ ran(R) Hengfeng Wei (hfwei@nju.edu.cn) 1-9 Set Theory (II): Relations 2019 年 12 月 03 日 16 / 51

5 Operations Hengfeng Wei hfweiinju.edu.cn1-9 Set Theory (II:Relations 2019年12月03日17/51
5 Operations Hengfeng Wei (hfwei@nju.edu.cn) 1-9 Set Theory (II): Relations 2019 年 12 月 03 日 17 / 51

Definition (Inverse) The inverse of R is the relation R-1={(a,b)|(b,a)∈R} Theorem (R-1)-1=R Definition (Restriction) The restriction of R to X is the relation Rx={(a,b)∈R|a∈X} Hengfeng Wei (hfweignju.edu.cn) 1-9 Set Theory (II):Relations 2019年12月03日18/51
Definition (Inverse) The inverse of R is the relation R −1 = {(a, b) | (b, a) ∈ R} Theorem (R −1 ) −1 = R Definition (Restriction) The restriction of R to X is the relation R|X = {(a, b) ∈ R | a ∈ X} Hengfeng Wei (hfwei@nju.edu.cn) 1-9 Set Theory (II): Relations 2019 年 12 月 03 日 18 / 51