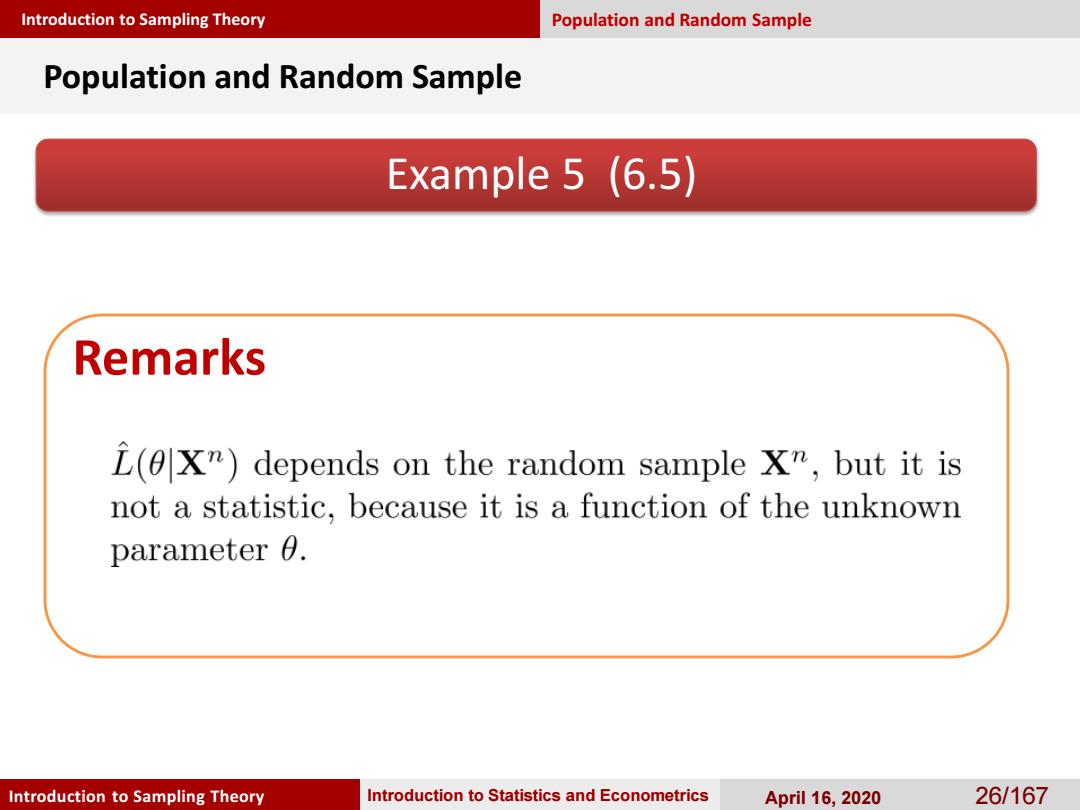
Introduction to Sampling Theory Population and Random Sample Population and Random Sample Example 5 (6.5) Remarks L(X")depends on the random sample X",but it is not a statistic,because it is a function of the unknown parameter 0. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 26/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 26/167 Remarks Example 5 (6.5) Population and Random Sample Population and Random Sample
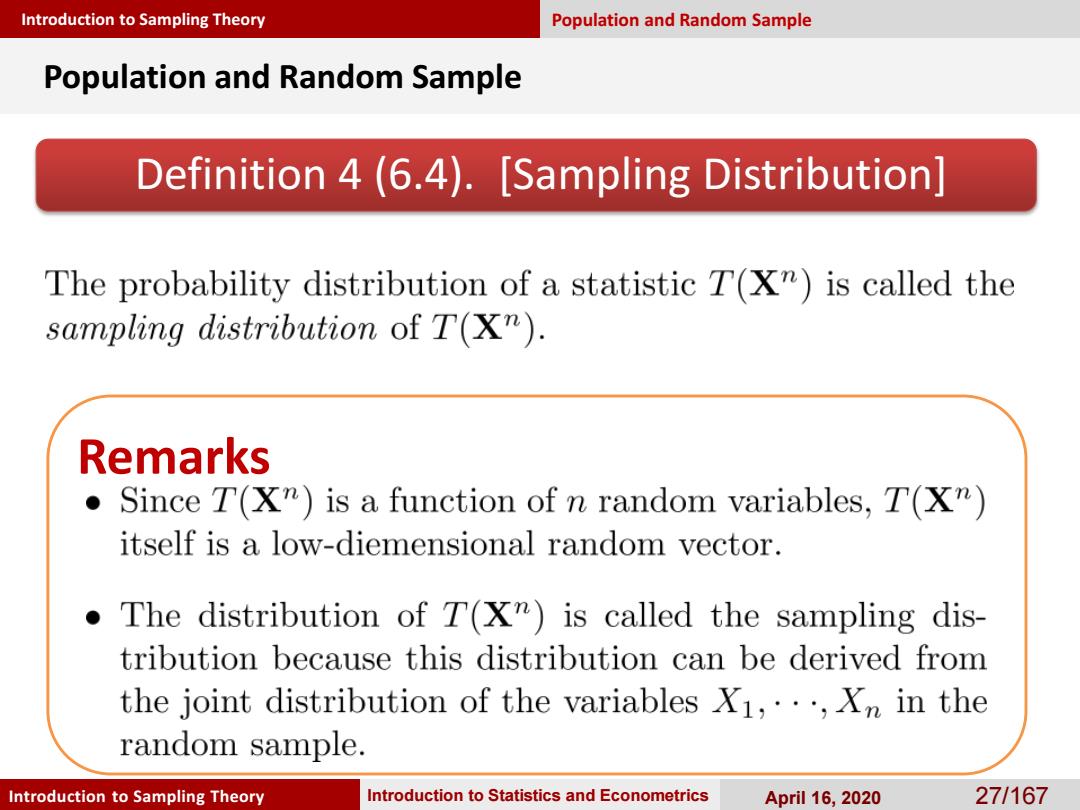
Introduction to Sampling Theory Population and Random Sample Population and Random Sample Definition 4(6.4).[Sampling Distribution] The probability distribution of a statistic T(X")is called the sampling distribution of T(X"). Remarks Since T(X")is a function of n random variables,T(X") itself is a low-diemensional random vector. The distribution of T(X")is called the sampling dis- tribution because this distribution can be derived from the joint distribution of the variables X1,...,Xn in the random sample. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 27/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 27/167 Definition 4 (6.4). [Sampling Distribution] Population and Random Sample Population and Random Sample Remarks

Introduction to Sampling Theory Population and Random Sample Population and Random Sample The sampling distribution of T(X")is different from the population distribution Fx(x).The latter is the marginal distribution of each Xi in an IID random sample X". The sampling distribution of a statistic T(X")plays a vital role in statistical inference.For example,it is needed to obtain critical values when constructing a confidence interval estimator and a hypothesis test statistic. T(X")can be viewed as a partition of the sample space of X".A random sample X"can generate many data sets x",each of which is called a sample point in the sample space of X".Let A(t)={x”:T(x)=t} be the collection of all sample points x"that satisfy the restriction T(x")=t.Then a single value of T(x")=t summarizes all sample points in A(t)which give the same value for T(x"). Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 28/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 28/167 Definition 4 (6.4). [Sampling Distribution] Population and Random Sample Population and Random Sample
CONTENTS 6.1 Population and Random Sample 6.2 Sampling Distribution of Sample Mean 6.3 Sampling Distribution of Sample Variance 6.4 Student's t-Distribution 6.5 Snedecor's F Distribution 6,6 Sufficient Statistics 6.7 Conclusion Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 29/167
Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 29/167 6.1 Population and Random Sample 6.2 Sampling Distribution of Sample Mean 6.3 Sampling Distribution of Sample Variance 6.4 Student’s t-Distribution 6.5 Snedecor's F Distribution 6.6 Sufficient Statistics 6.7 Conclusion CONTENTS
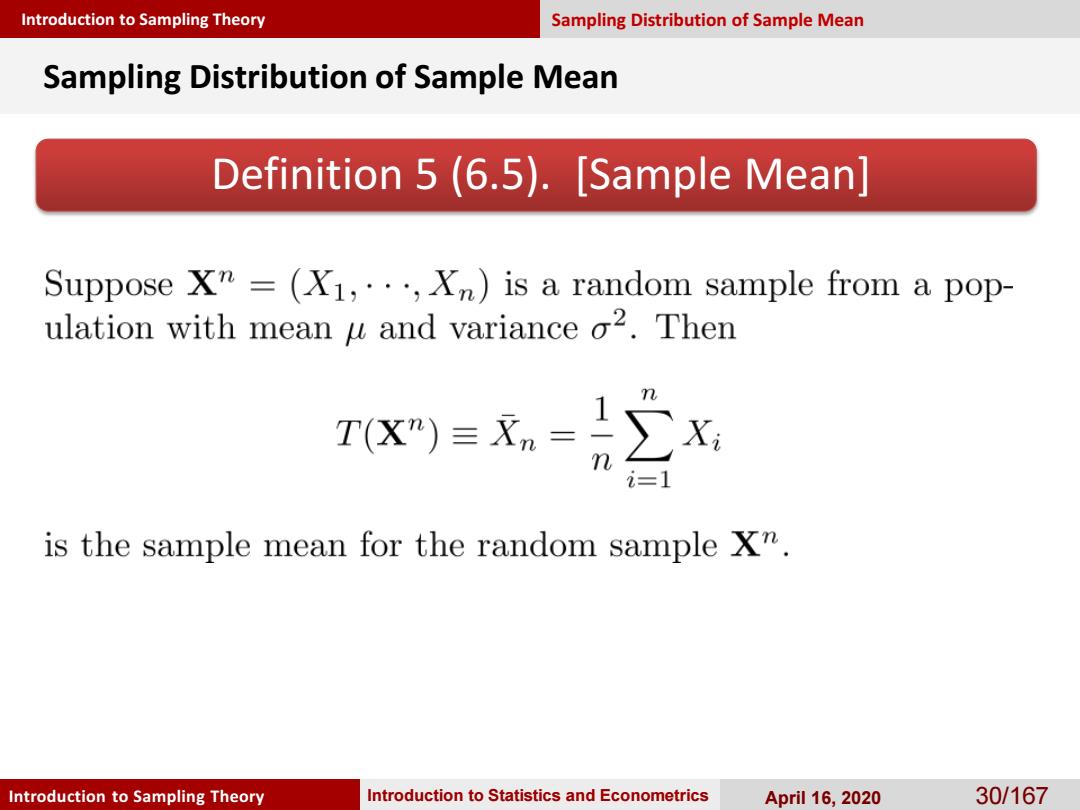
Introduction to Sampling Theory Sampling Distribution of Sample Mean Sampling Distribution of Sample Mean Definition 5(6.5).[Sample Mean] Suppose XT=(X1,..,Xn)is a random sample from a pop- ulation with mean u and variance o2.Then TX)=X-∑X: 2=1 is the sample mean for the random sample X". Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 30/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 30/167 Definition 5 (6.5). [Sample Mean] Sampling Distribution of Sample Mean Sampling Distribution of Sample Mean