
Introduction to Sampling Theory Population and Random Sample Population and Random Sample Remarks: The function T()is a mapping from the n-dimensional sample space of X"to a low-dimensional Euclidean space. .A statistic T(X")does not involve any unknown parameter.It is entirely a function of random sample X".Given any data set x",we can obtain a real-valued number or vector for the statistic T(X"). Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 21/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 21/167 Population and Random Sample Population and Random Sample Remarks:
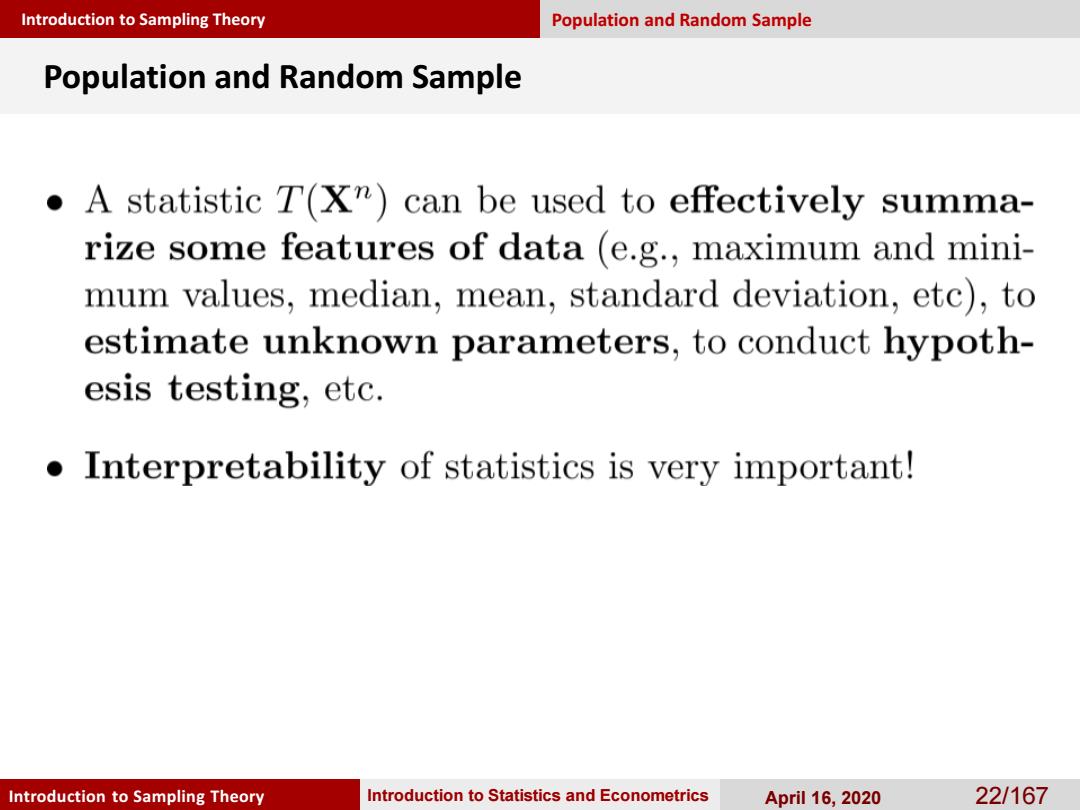
Introduction to Sampling Theory Population and Random Sample Population and Random Sample .A statistic T(X")can be used to effectively summa- rize some features of data (e.g.,maximum and mini- mum values,median,mean,standard deviation,etc),to estimate unknown parameters,to conduct hypoth- esis testing,etc. Interpretability of statistics is very important! Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 22/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 22/167 Population and Random Sample Population and Random Sample
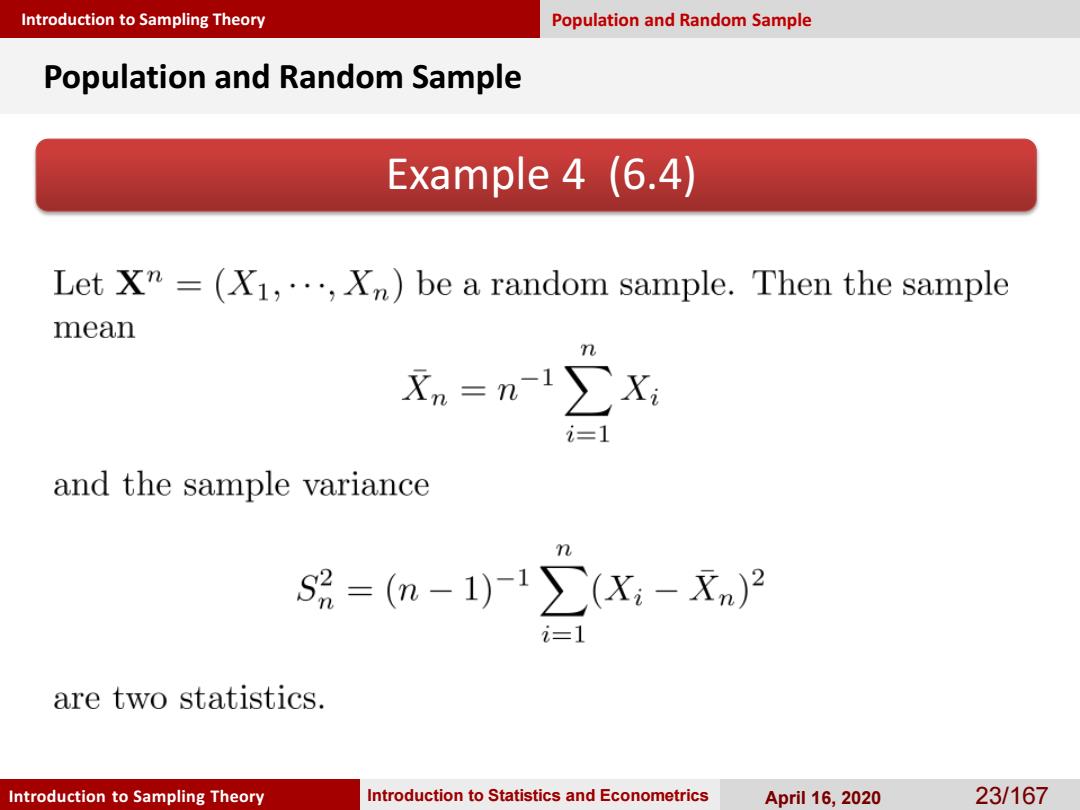
Introduction to Sampling Theory Population and Random Sample Population and Random Sample Example 4 (6.4) Let X"=(X1,...,Xn)be a random sample.Then the sample mean Xm=n1∑X: 2=1 and the sample variance S2=(n-1)1∑(X:-Xn)2 1 are two statistics. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 23/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 23/167 Example 4 (6.4) Population and Random Sample Population and Random Sample

Introduction to Sampling Theory Population and Random Sample Population and Random Sample Question:n and S2 can be used to estimate x and of the population distribution Fx().Why are n and S? ”good”estimators of ux and o respectively? We will develop various concepts to measure the close- ness of an estimator to the parameter of interest in Chap- ters 7 and 8. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 24/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 24/167 Population and Random Sample Population and Random Sample

Introduction to Sampling Theory Population and Random Sample Population and Random Sample Example 5 (6.5) Let X"=(X1,...,Xn)be an IID random sample from the population f(x,0),where 0 is some unknown parameter.Then the logarithm of the joint PMF/PDF of X" i(0X")=lnf(X,0) i=1 ∑lnf(X,) i=1 is called the log-likelihood function of 0,conditional on the random sample Xm. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 25/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 25/167 Example 5 (6.5) Population and Random Sample Population and Random Sample