
hand,if B moves first,its profit is 55.If it moves second,its profit is 45,a differene of 10,and it thus would be willing topend upto10 for the option of announcing first. Once Firm A realizes that Firm B is willing to spend something on the option of announcing first,then the value of the option decreases for Firm A.because if both firms were to invest both firms would choose to produce the high-quality system. which gives them both a lower pavoff.Firm A should not spend money to speed up the introduction of its product in any case if b goes first and chooses high then A instead of the50it ent high also This is best for B also because if B goes high and A low,Bends up with 55 instead of 40.so even ifit spends the 10 it is still ahead by 5.A should let B go first.Finally. note that even though B is better off if A goes first and chooses high(45 vs.40).B is still best off if it es first.Overall it is worthwhile for B to spend the money and announce but it is not worthwhile for A tospend any money 4.Two firms are in the chocolate market.Each can choose togo for the high end of the market (high quality)or the low end (low quality). Resulting profits are given by the following payoff matrix: Firm2 High Low 20,30 900,600 Firm 1 High 100,800 50,50 What outcomes,if any,are Nash equilibria? A Nash equilibrium exists when neither party has an inentive to alter its strategy,taking the other's strategy as given.If Firm 2 chooses Low and Firm I chooses High,neither will have an incentive to change (100>20 for Firm 1 and 800>50 for Firm 2).If Firm 2 chooses High and Firm 1 chooses low.neither will have an incentive to change (900>50 for Firm 1 and 600>30 for Firm 2).Both outoomes are Nash equilibria.Both firms choosing low is nota Nash equilibrium because,for example,if Firm chooses low then firm 2 is better off by switching to high since 600 is greater than -30. If the manager of each firm is conservative and each follows a maximin strategy,what will be the outcome? If Firm 1 chooses Low.its worst pavoff-20.would occur if Firm 2 chooses Low.If Firm 1 chooses High its worst payoff 50,would occur if Firm chooses High. .Therefore,with a conservative maximin strategy,Firm l
hand, if B moves first, its profit is 55. If it moves second, its profit is 45, a difference of 10, and it thus would be willing to spend up to 10 for the option of announcing first. Once Firm A realizes that Firm B is willing to spend something on the option of announcing first, then the value of the option decreases for Firm A, because if both firms were to invest both firms would choose to produce the high-quality system, which gives them both a lower payoff. Firm A should not spend money to speed up the introduction of its product in any case. If B goes first and chooses high then A can choose low and end up with 55 instead of the 50 it would get if it went high also. This is best for B also because if B goes high and A low, B ends up with 55 instead of 40, so even if it spends the 10 it is still ahead by 5. A should let B go first. Finally, note that even though B is better off if A goes first and chooses high (45 vs. 40), B is still best off if it goes first. Overall, it is worthwhile for B to spend the money and announce but it is not worthwhile for A to spend any money. 4. Two firms are in the chocolate market. Each can choose to go for the high end of the market (high quality) or the low end (low quality). Resulting profits are given by the following payoff matrix: Firm 2 Low High Low -20, -30 900, 600 Firm 1 High 100, 800 50, 50 a. What outcomes, if any, are Nash equilibria? A Nash equilibrium exists when neither party has an incentive to alter its strategy, taking the other’s strategy as given. If Firm 2 chooses Low and Firm 1 chooses High, neither will have an incentive to change (100 > -20 for Firm 1 and 800 > 50 for Firm 2). If Firm 2 chooses High and Firm 1 chooses Low, neither will have an incentive to change (900 > 50 for Firm 1 and 600 > -30 for Firm 2). Both outcomes are Nash equilibria. Both firms choosing low is not a Nash equilibrium because, for example, if Firm 1 chooses low then firm 2 is better off by switching to high since 600 is greater than -30. b. If the manager of each firm is conservative and each follows a maximin strategy, what will be the outcome? If Firm 1 chooses Low, its worst payoff, -20, would occur if Firm 2 chooses Low. If Firm 1 chooses High, its worst payoff, 50, would occur if Firm 2 chooses High. Therefore, with a conservative maximin strategy, Firm 1
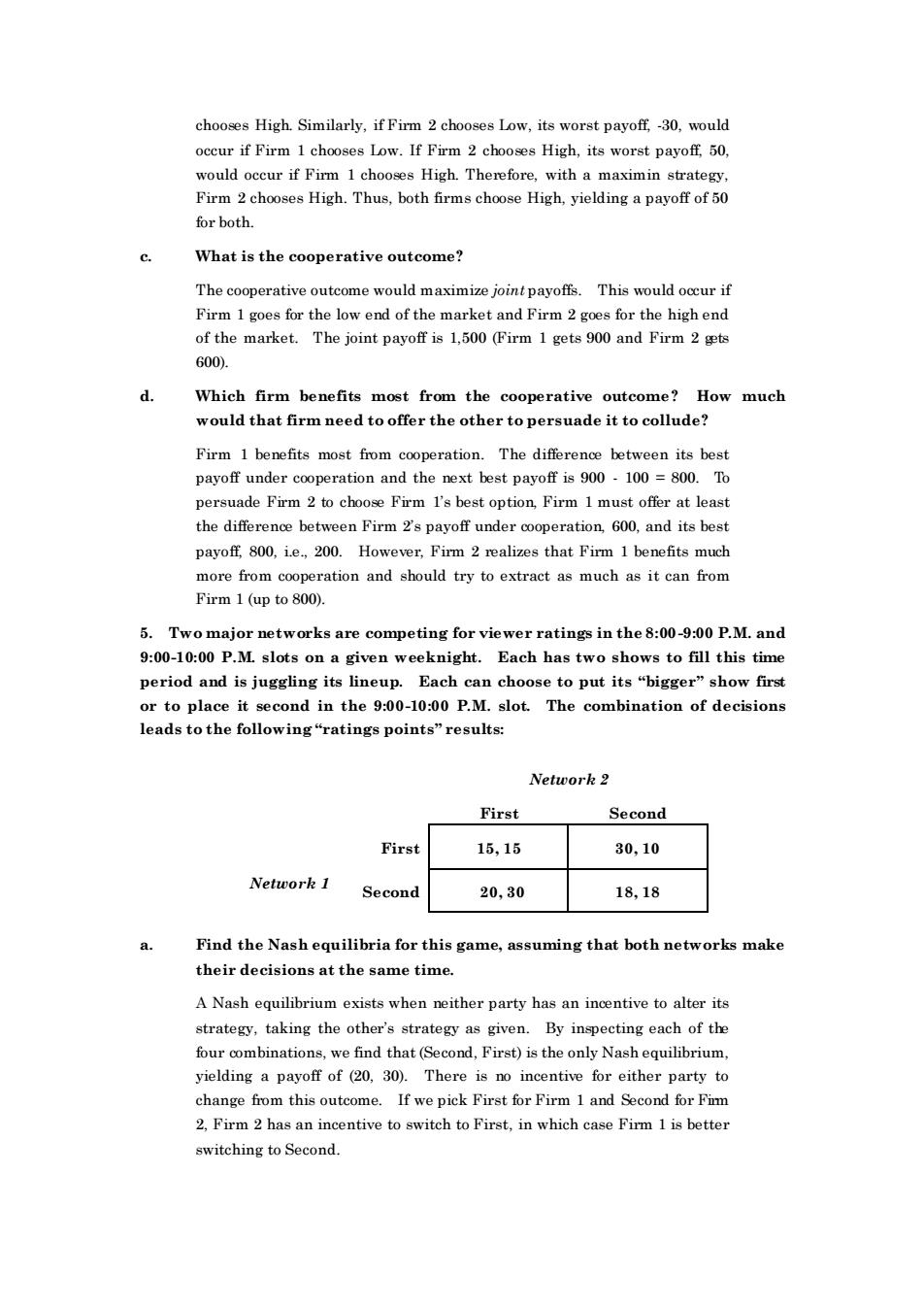
chooses High.Similarly,if Firm 2 chooses Low,its worst payoff,-30,would occur if Firm 1 chooses Low.If Firm 2 chooses High,its worst payoff50. would oceur if Firm I chooses High.Therefore, with a maximin strategy Firm 2 chooses High.Thus,both firms choose High,vielding a payoff of 50 for both. What is the cooperative outcome? The cooperative outcome would maximize joint payoffs.This would occur if Firm 1goes for the low end of the market and Firm 2goes for the highend The payoff is 1.500 (Firm 1gets 90 and Firm 2 gts G00 Which firm benefits most from the cooperative outcome? How much would that firm need to offer the other to persuade it to collude? Firm 1 benefits most from cooperation.The difference between its best payoff under cooperation and the next best payoff is 900.100 =800.To persuade Firm 2 to cboose firm l's best option.Firm 1 must offer at least the differen between Firm2s payof under its best payoff 800.However,Firm 2 realizes that Firm 1 benefits much more from cooperation and should try to extract as much as it can from Firm 1 (up to 800). 5.Two major networks are competing for viewer ratings in the8:00-9:00 P.M.and 9:00-10:00 P.M.slots on a given weeknight.Each has two shows to fill this time period and is juggling its lin Each can choose to put its"bigger"show first or to plac it second in the:0-10:00P.M. slot. The combination of decisions leads to the following"ratings points"results Netuork 2 First Second First 15,15 30,10 Second 20,30 18,18 Find the Nash equilibria for this game,assuming that both networks make their decisions at the same time A Nash equilibrium exists when neither party has an incentive to alter its strategy,taking the other's strategy as given.By inspecting each of the four combinations,we find that(Second,First)is the only Nash equilibrium. yielding a payoff of (20,30).There is no incentive for either party to change from this outcome.If we pick First for Firm 1 and Second for Fim 2.Firm 2 has an incentive to switch to First.in which case Firm 1is better switching to Second
chooses High. Similarly, if Firm 2 chooses Low, its worst payoff, -30, would occur if Firm 1 chooses Low. If Firm 2 chooses High, its worst payoff, 50, would occur if Firm 1 chooses High. Therefore, with a maximin strategy, Firm 2 chooses High. Thus, both firms choose High, yielding a payoff of 50 for both. c. What is the cooperative outcome? The cooperative outcome would maximize joint payoffs. This would occur if Firm 1 goes for the low end of the market and Firm 2 goes for the high end of the market. The joint payoff is 1,500 (Firm 1 gets 900 and Firm 2 gets 600). d. Which firm benefits most from the cooperative outcome? How much would that firm need to offer the other to persuade it to collude? Firm 1 benefits most from cooperation. The difference between its best payoff under cooperation and the next best payoff is 900 - 100 = 800. To persuade Firm 2 to choose Firm 1’s best option, Firm 1 must offer at least the difference between Firm 2’s payoff under cooperation, 600, and its best payoff, 800, i.e., 200. However, Firm 2 realizes that Firm 1 benefits much more from cooperation and should try to extract as much as it can from Firm 1 (up to 800). 5. Two major networks are competing for viewer ratings in the 8:00-9:00 P.M. and 9:00-10:00 P.M. slots on a given weeknight. Each has two shows to fill this time period and is juggling its lineup. Each can choose to put its “bigger” show first or to place it second in the 9:00-10:00 P.M. slot. The combination of decisions leads to the following “ratings points” results: Network 2 First Second First 15, 15 30, 10 Network 1 Second 20, 30 18, 18 a. Find the Nash equilibria for this game, assuming that both networks make their decisions at the same time. A Nash equilibrium exists when neither party has an incentive to alter its strategy, taking the other’s strategy as given. By inspecting each of the four combinations, we find that (Second, First) is the only Nash equilibrium, yielding a payoff of (20, 30). There is no incentive for either party to change from this outcome. If we pick First for Firm 1 and Second for Firm 2, Firm 2 has an incentive to switch to First, in which case Firm 1 is better switching to Second