
ADVANCED MACROECONOMICS Lecture 4 New Growth Theory 电子科大经管学院马捷
ADVANCED MACROECONOMICS Lecture 4 New Growth Theory 电子科大经管学院 马捷 1

Outline ·AK model 0 Model with human capital Learning by doing The Romer model Aghion-Howitt model 电子科大经管学院马捷 2 元元元元元
Outline AK model Model with human capital Learning by doing The Romer model Aghion-Howitt model 电子科大经管学院 马捷 2
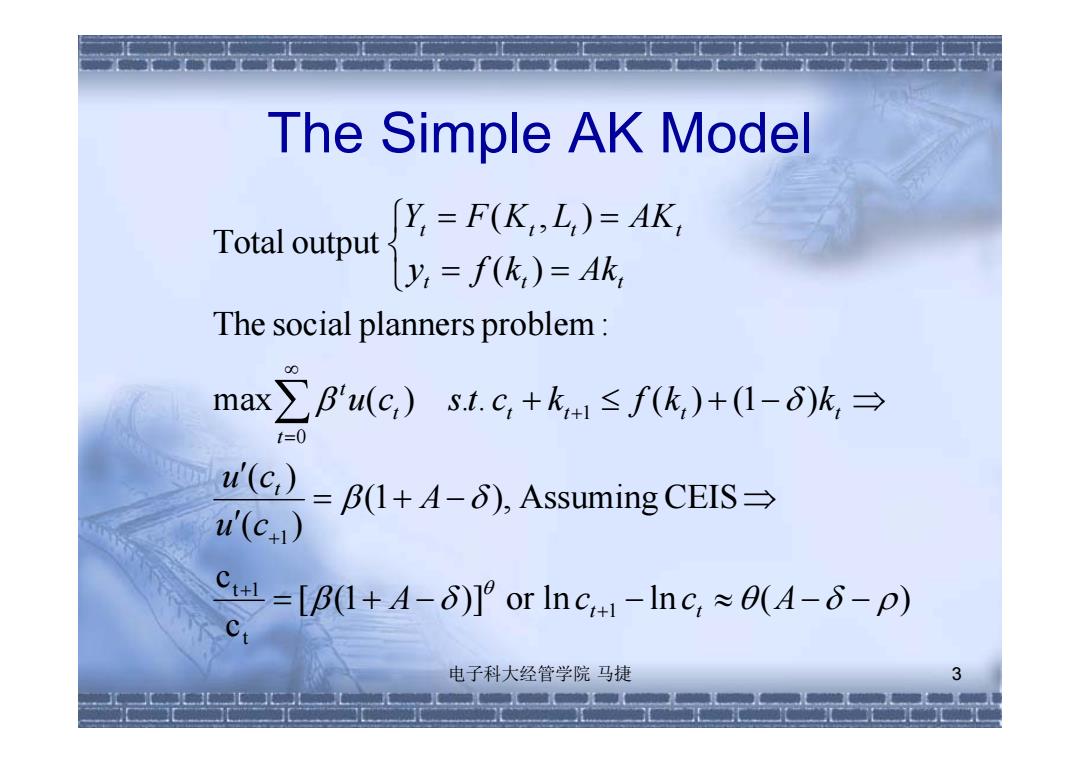
The Simple AK Model Y=F(KL)=AK Total output y=f(k )Ak The social planners problem: max∑B'u(c,)s.t.c,+k+1≤f(k,)+(1-o)k,→ t=0 u'(c)B(1+A-5),Assuming CEIS W'(c) cL-[BI+A-δ)°or Ine,1-lnc,≈(A-6-p) C 电子科大经管学院马捷 3 己元元
The Simple AK Model 电子科大经管学院 马捷 3 [ ( 1 )] or ln ln ( ) c c ( 1 ), Assuming CEIS ( ) ( ) max ( ) . . ( ) ( 1 ) The social planners problem: ( ) ( , ) Total output 1 t t 1 1 1 0 A c c A A u c u c u c s t c k f k k y f k Ak Y F K L AK t t t t t t t t t t t t t t t t t

The resource constraint c,+k+l=(l+A-δ)k,→ G=0-0+A-k→1-知= k+1=s(1+A-δ)k k,y To ensure perpetual growth,we thus need B(1+A-6)>1orA-6>p Otherwise,the economy would shrink at a constant rate to 0 电子科大经管学院马捷
电子科大经管学院 马捷 4 at a constant rate to 0 Otherwise,the economy would shrink (1 ) 1or To ensure perpetual growth, we thus need (1 ) (1 )(1 ) The resource constraint (1 ) 1 1 1 1 1 A A y y k k c c k s A k c s A k c k A k t t t t t t t t t t t t t

C,+k+1=(1+A-δ)k,→ +k1=1+A-0) k,k k=9 K L=[B1+A-δ)]→s=B(1+A-δ)91 C C,=(1-S1+A-δ)k, To ensures∈(0,1),we impose B(l+A-6)-<1 电子科大经管学院马捷 5
电子科大经管学院 马捷 5 To ensure s (0,1), we impose ( 1 ) 1 ( 1 ) ( 1 )( 1 ) [ ( 1 )] ( 1 ) ( 1 ) 1 1 1 1 1 1 A s A c s A k A c c k k A k k k c c k A k t t t t t t t t t t t t t