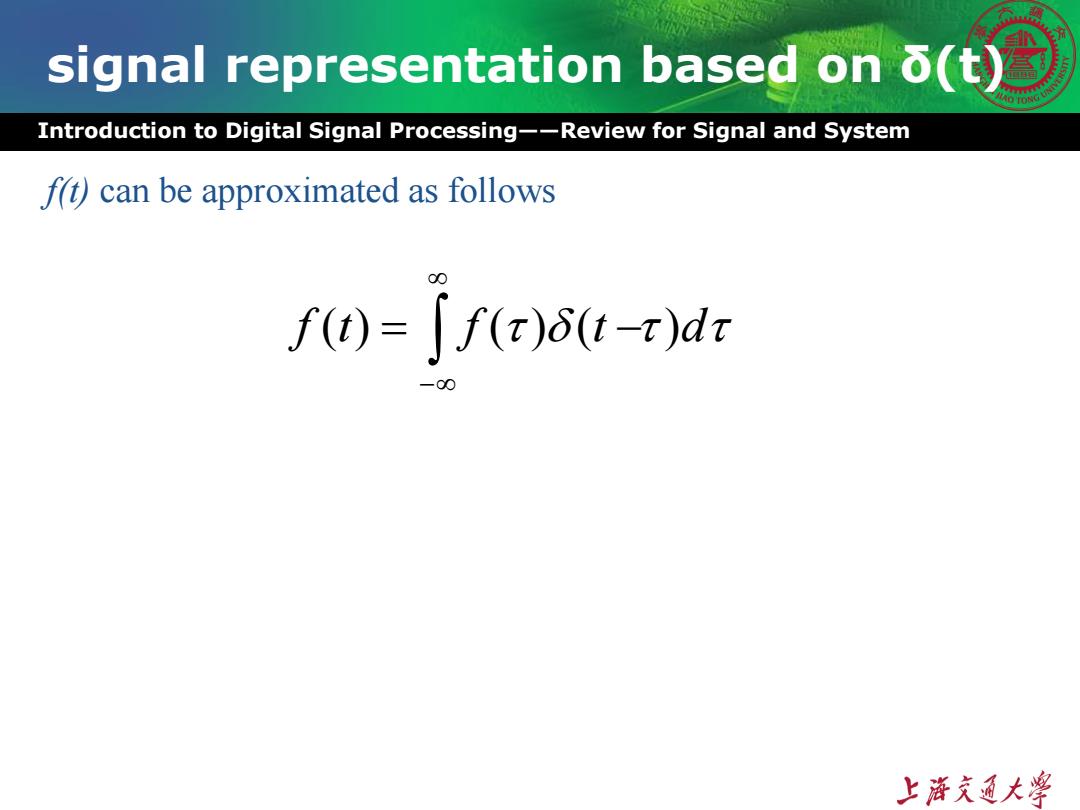
signal representation based on 6(t) Introduction to Digital Signal Processing--Review for Signal and System ft)can be approximated as follows f)=∫f(r)u-r)dr 上游充通大
Introduction to Digital Signal Processing——Review for Signal and System signal representation based on δ(t) f(t) can be approximated as follows f (t) f ( ) (t )d
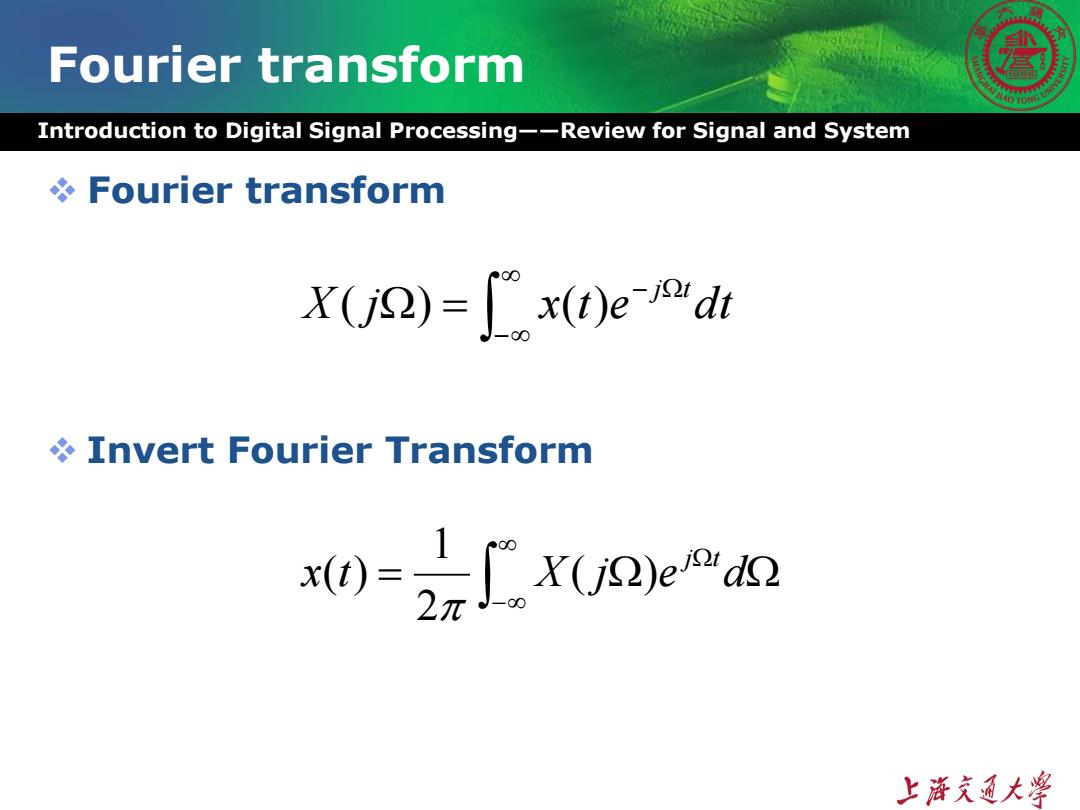
Fourier transform Introduction to Digital Signal Processing--Review for Signal and System Fourier transform X(j)="x(t)e-dt Invert Fourier Transform 02aJXnen 上游充通大
Introduction to Digital Signal Processing——Review for Signal and System Fourier transform Invert Fourier Transform Fourier transform X j x t e dt j t ( ) ( ) x t X j e d j t ( ) 2 1 ( )
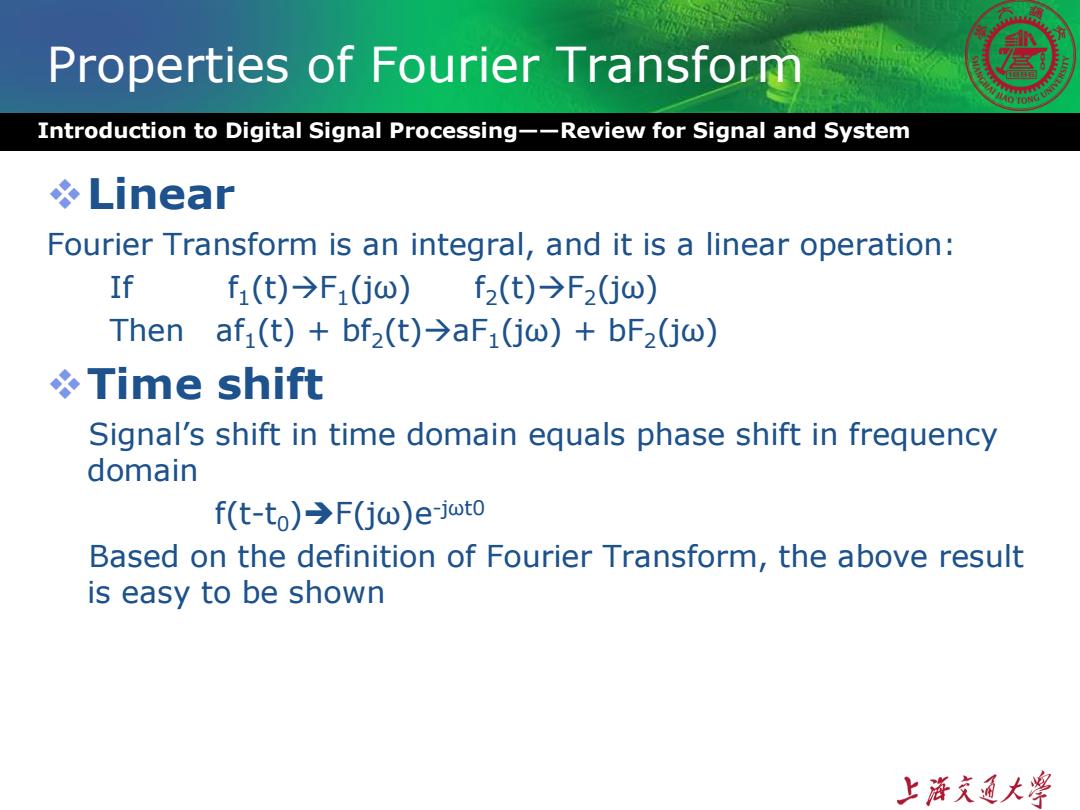
Properties of Fourier Transform HAO TO Introduction to Digital Signal Processing--Review for Signal and System Linear Fourier Transform is an integral,and it is a linear operation: If f1(t)→F1jω) f2(t)→F2(j) Then af (t)+bf2(t)>aF(jw)+bF2(j@) Time shift Signal's shift in time domain equals phase shift in frequency domain f(t-to)→F(jω)e-jwt0 Based on the definition of Fourier Transform,the above result is easy to be shown 上游充通大
Introduction to Digital Signal Processing——Review for Signal and System Properties of Fourier Transform Linear Fourier Transform is an integral, and it is a linear operation: If f1 (t)F1 (jω) f2 (t)F2 (jω) Then af1 (t) + bf2 (t)aF1 (jω) + bF2 (jω) Time shift Signal’s shift in time domain equals phase shift in frequency domain f(t-t0 )F(jω)e-jωt0 Based on the definition of Fourier Transform, the above result is easy to be shown