
Parameter Estimation and Evaluation Introduction to Hypothesis Testing Introduction to Hypothesis Testing There exists a tradeoff between Type I errors and Type II errors given any sample size n.For any given n,if the critical region C shrinks,the probability of making Type I error deceases,but the probability of making Type II error increases.Similarly,if the critical region C increases, the Type II error B(0)decreases,but Type I error a(0) increases. 0 Usually,hypothesis tests are evaluated and compared through their probabilities of making mistakes.The classical ap- proach to hypothesis testing is to bound the probability of Type I error by some value a E(0,1)over all values of 0 in 9o and to try to find a test that minimizes the probability of a Type II error over all values ofθin曰A. Hypothesis Testing Introduction to Statistics and Econometrics July7,2020 26/110
Parameter Estimation and Evaluation Hypothesis Testing Introduction to Statistics and Econometrics July 7, 2020 26/110 Introduction to Hypothesis Testing Introduction to Hypothesis Testing

Parameter Estimation and Evaluation Introduction to Hypothesis Testing Introduction to Hypothesis Testing Definition 7(9.7)[Uniformly Most Powerful Test] Let T be a class of tests for testing Ho 0 E o versus HA: 0∈ΘA.A test T(X)in class T,with power functionπ(g), is a uniformly most powerful test over T ifπ(O)≥元(O)for all 0∈ΘA,where元(θ)is the power function of any other test G(XT)in class T. Hypothesis Testing Introduction to Statistics and Econometrics July7,2020 27/110
Parameter Estimation and Evaluation Hypothesis Testing Introduction to Statistics and Econometrics July 7, 2020 27/110 Introduction to Hypothesis Testing Introduction to Hypothesis Testing Definition 7 (9.7) [Uniformly Most Powerful Test]
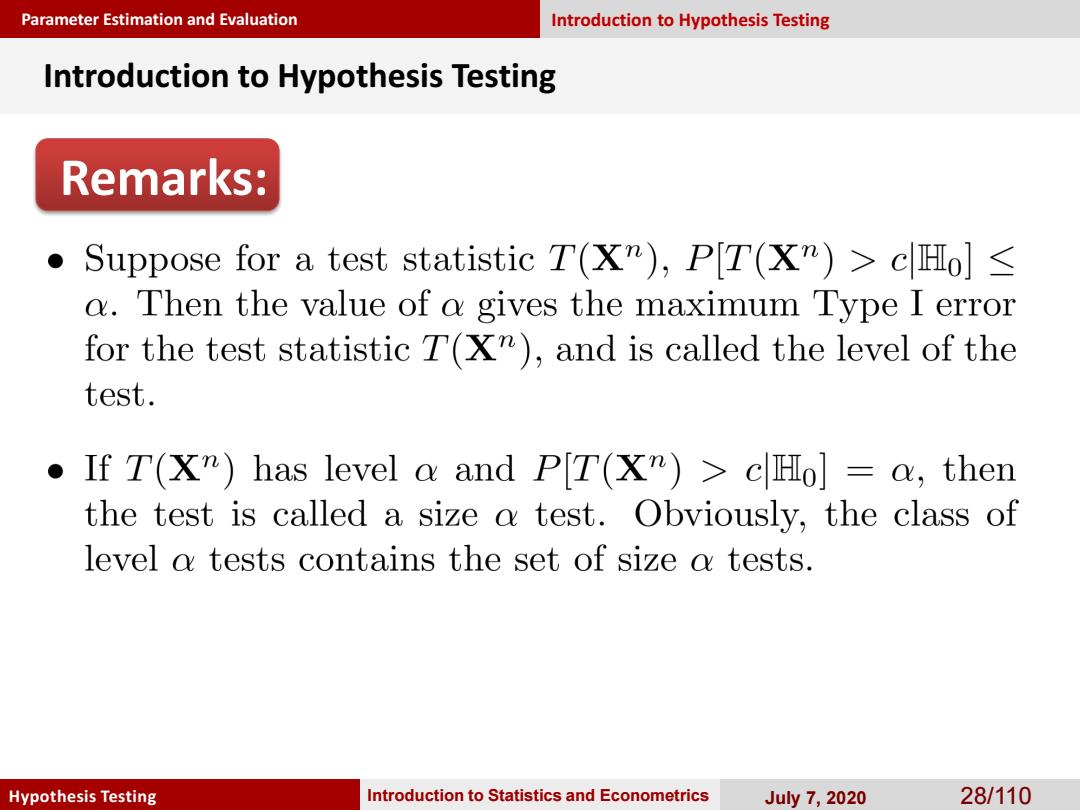
Parameter Estimation and Evaluation Introduction to Hypothesis Testing Introduction to Hypothesis Testing Remarks: Suppose for a test statistic T(Xm),PT(X")>cHo< a.Then the value of a gives the maximum Type I error for the test statistic T(X"),and is called the level of the test. .If T(X")has level a and PT(X")>cHo=a,then the test is called a size a test.Obviously,the class of level a tests contains the set of size a tests. Hypothesis Testing Introduction to Statistics and Econometrics Juy7,2020 28/110
Parameter Estimation and Evaluation Hypothesis Testing Introduction to Statistics and Econometrics July 7, 2020 28/110 Introduction to Hypothesis Testing Introduction to Hypothesis Testing Remarks:
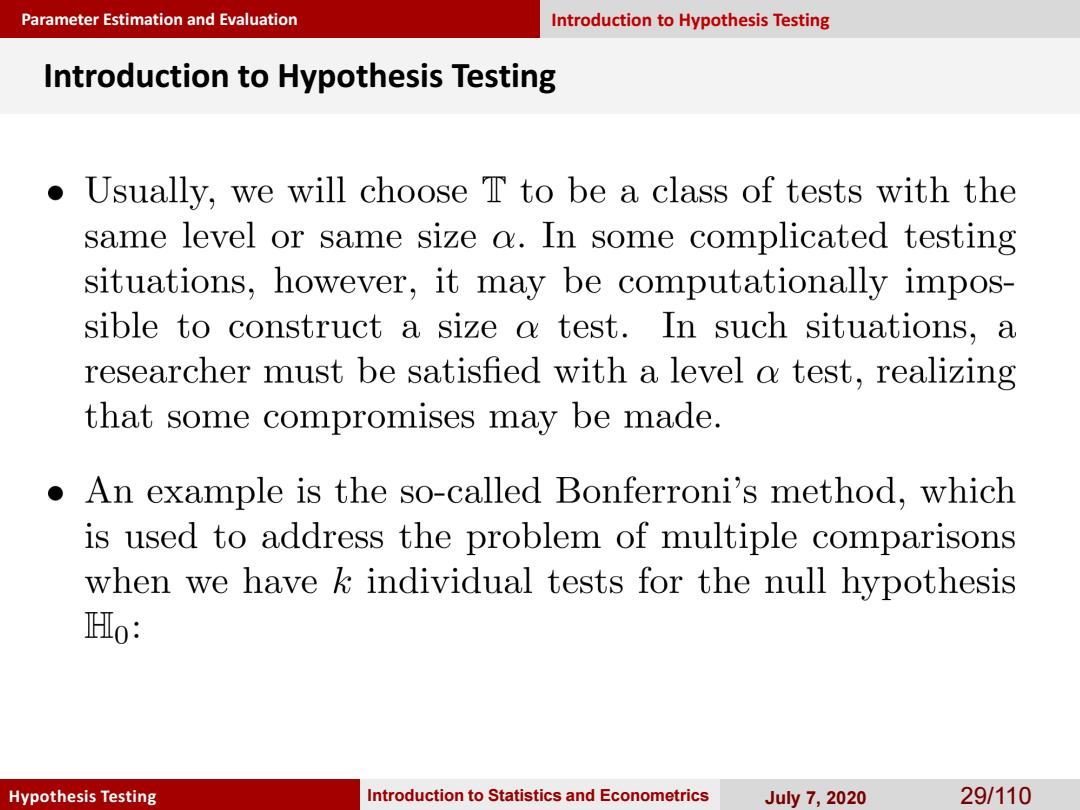
Parameter Estimation and Evaluation Introduction to Hypothesis Testing Introduction to Hypothesis Testing Usually,we will choose T to be a class of tests with the same level or same size a.In some complicated testing situations,however,it may be computationally impos- sible to construct a size a test.In such situations,a researcher must be satisfied with a level a test,realizing that some compromises may be made. .An example is the so-called Bonferroni's method,which is used to address the problem of multiple comparisons when we have k individual tests for the null hypothesis Ho: Hypothesis Testing Introduction to Statistics and Econometrics Juy7,2020 29/110
Parameter Estimation and Evaluation Hypothesis Testing Introduction to Statistics and Econometrics July 7, 2020 29/110 Introduction to Hypothesis Testing Introduction to Hypothesis Testing Remarks:

Parameter Estimation and Evaluation Introduction to Hypothesis Testing Introduction to Hypothesis Testing Suppose for each i∈{1,··,k},T(Xn)is a level a/k test for Ho,namely PT,(X")>cHo≤天, i=1,·,k, where ci is a critical value for Ti(X"). Hypothesis Testing Introduction to Statistics and Econometrics Juy7,2020 30/110
Parameter Estimation and Evaluation Hypothesis Testing Introduction to Statistics and Econometrics July 7, 2020 30/110 Introduction to Hypothesis Testing Introduction to Hypothesis Testing Remarks: