
HE =He(ri)+He-i(ri,Rjo) HE=∑p)2/(2m)+()∑i∑ir[e2Ir-rrl-∑∑lZ2Irr-Ro(i≠i') >Replace each electron momentum with its quantumoperator: Pi=-ihVi(everywhere in He!) Approximation #3 The Mean Field or One Electron Approximation For He:Make the Mean Field Approximation:Every electron experiences the same average potential due to coulomb repulsion plus coulomb attraction. The many electron Hamiltonian is replaced by a one electron Hamiltonian: Hie=(p)2/(2m)+V(r),p=-ihV In general,this is still a very complex,highly computational problem! V(r)=Effective Potential=Average potential ofone electron interacting with all others+all the ions V(r)is periodic has the lattice symmetry! Given V(r),solve the One Electron Schrodinger Equation:Hlewn=Enwn n=eigenvalue label(band index),wn=one electron wavefunction
Approximation #3 The Mean Field or One Electron Approximation For HE: Make the Mean Field Approximation: Every electron experiences the same average potential due to coulomb repulsion plus coulomb attraction. The many electron Hamiltonian is replaced by a one electron Hamiltonian: H1e = (p)2 /(2mo ) + V(r), p -iħ V(r) Effective Potential Average potential of one electron interacting with all others + all the ions V(r) is periodic & has the lattice symmetry! Given V(r), solve the One Electron Schrödinger Equation: H1eψn = Enψn n = eigenvalue label (band index) , ψn = one electron wavefunction HE = He (ri ) + He-i (ri ,Rjo) HE = ∑i (pi ) 2 /(2mi ) + (½)∑i∑i´ [e2 /|ri -ri´ |] - ∑i∑ j [Zje 2 /|ri -Rjo|] (i i´) Replace each electron momentum with its quantum operator: pi -iħi (everywhere in HE!) In general, this is still a very complex, highly computational problem!
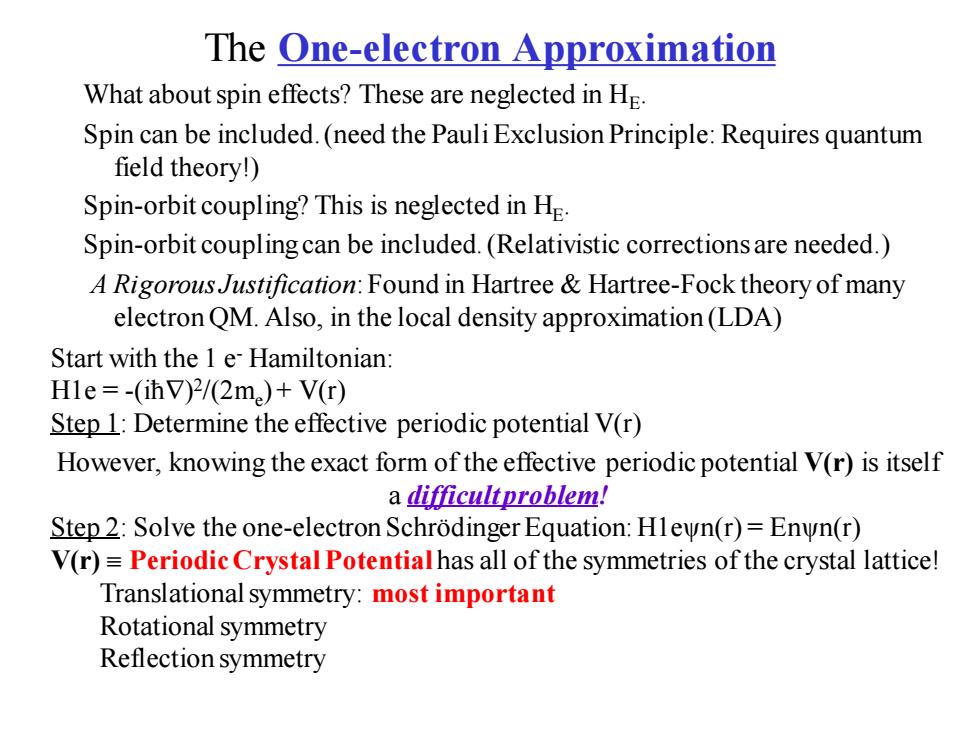
The One-electron Approximation What about spin effects?These are neglected in He. Spin can be included.(need the Pauli Exclusion Principle:Requires quantum field theory!) Spin-orbit coupling?This is neglected in He Spin-orbit coupling can be included.(Relativistic corrections are needed.) A Rigorous Justification:Found in Hartree Hartree-Fock theory of many electron QM.Also,in the local density approximation(LDA) Start with the 1 e Hamiltonian: Hle=-(ihV)2/(2m)+V(r) Step 1:Determine the effective periodic potential V(r) However,knowing the exact form of the effective periodic potential V(r)is itself a difficultproblem! Step 2:Solve the one-electron Schrodinger Equation:Hlewn(r)=Enwn(r) V(r)=Periodic Crystal Potentialhas all of the symmetries of the crystal lattice! Translational symmetry:most important Rotational symmetry Reflection symmetry
The One-electron Approximation What about spin effects? These are neglected in HE . Spin can be included. (need the Pauli Exclusion Principle: Requires quantum field theory!) Spin-orbit coupling? This is neglected in HE . Spin-orbit coupling can be included. (Relativistic corrections are needed.) A Rigorous Justification: Found in Hartree & Hartree-Fock theory of many electron QM. Also, in the local density approximation (LDA) Start with the 1 e- Hamiltonian: H1e = -(iħ) 2 /(2me ) + V(r) Step 1: Determine the effective periodic potential V(r) However, knowing the exact form of the effective periodic potential V(r) is itself a difficult problem! Step 2: Solve the one-electron Schrödinger Equation: H1eψn(r) = Enψn(r) V(r) Periodic Crystal Potential has all of the symmetries of the crystal lattice! Translational symmetry: most important Rotational symmetry Reflection symmetry

a point worthy of attention: scientists establish the band theory with crystalline solid and from the point vies of periodic potential at first.But the introduction of periodic potential is to simplify the problem.In fact,periodic potential is not the necessary conditions for the electronic band structure,which has been confirmed to be the same as amorphous solids,eg,electron also has a band structure. Band formation results from the interaction between atoms, and does not depend on the way of atom bonding,the arrangement of atoms with translational symmetry is not the necessary conditions for the band formation
scientists establish the band theory with crystalline solid and from the point vies of periodic potential at first. But the introduction of periodic potential is to simplify the problem. In fact, periodic potential is not the necessary conditions for the electronic band structure, which has been confirmed to be the same as amorphous solids , eg, electron also has a band structure. Band formation results from the interaction between atoms, and does not depend on the way of atom bonding, the arrangement of atoms with translational symmetry is not the necessary conditions for the band formation. a point worthy of attention: