
问题4: 线性规划问题中的不等 式能不能用严格的大于 或小于?
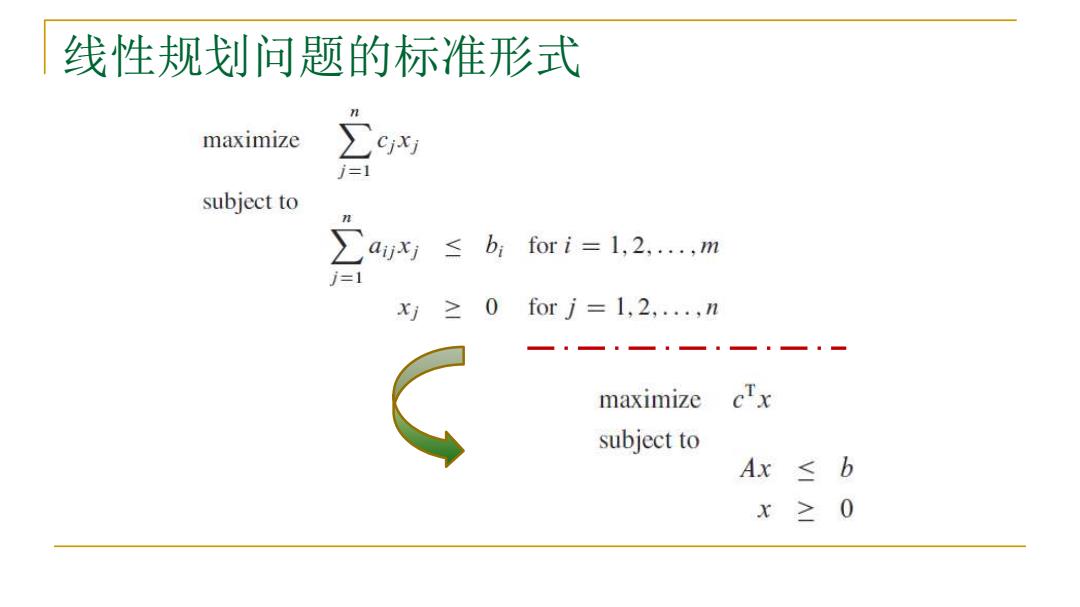
线性规划问题的标准形式 maximize j=1 subject to ax≤bi for i=1,2,,m j=1 x≥0forj=1,2,..,n maximize cTx subject to Ax ≤b x≥0
线性规划问题的标准形式

minimize -2x1+3x2 subject to x1+x2 =7 1- 2x2 4 X1 ≥0 问题5: 为什么说这不是“标准形式
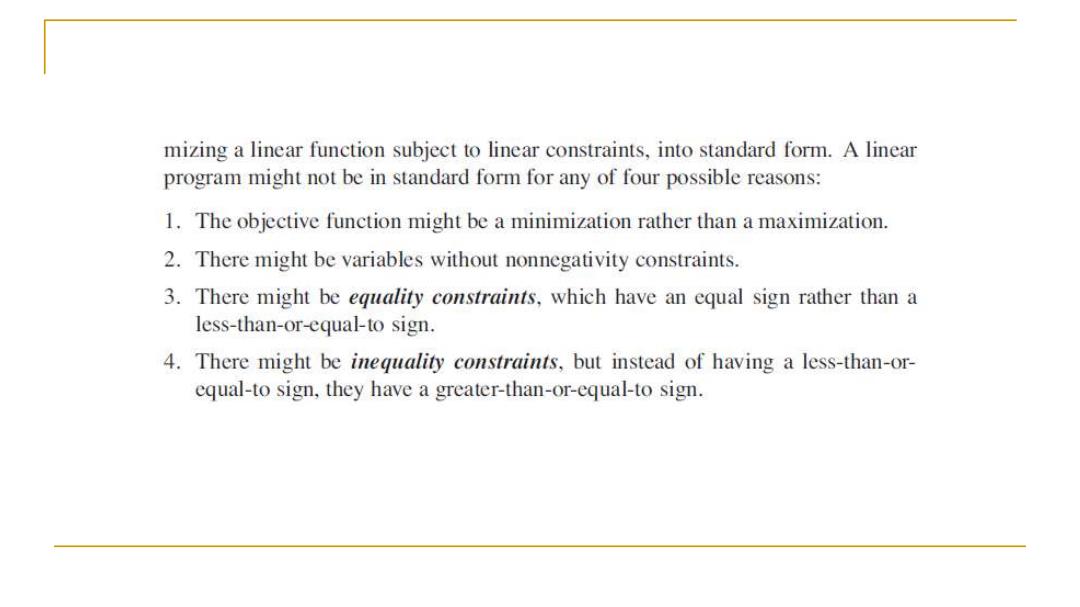
mizing a linear function subject to linear constraints,into standard form.A linear program might not be in standard form for any of four possible reasons: 1.The objective function might be a minimization rather than a maximization. 2.There might be variables without nonnegativity constraints. 3.There might be equality constraints,which have an equal sign rather than a less-than-or-equal-to sign. 4.There might be inequality constraints,but instead of having a less-than-or- equal-to sign,they have a greater-than-or-equal-to sign

minimize -2x1+3x2 maximize 2x1 3x2 subject to subject to x1+ X2 =7 x1+ X2 =7 x1-2x2 4 → x1-2x2 ≤4 x1 0. X1 ≥0 问题6: 如何将它转化为标准形式?这 两个线性规划“一样”吗?