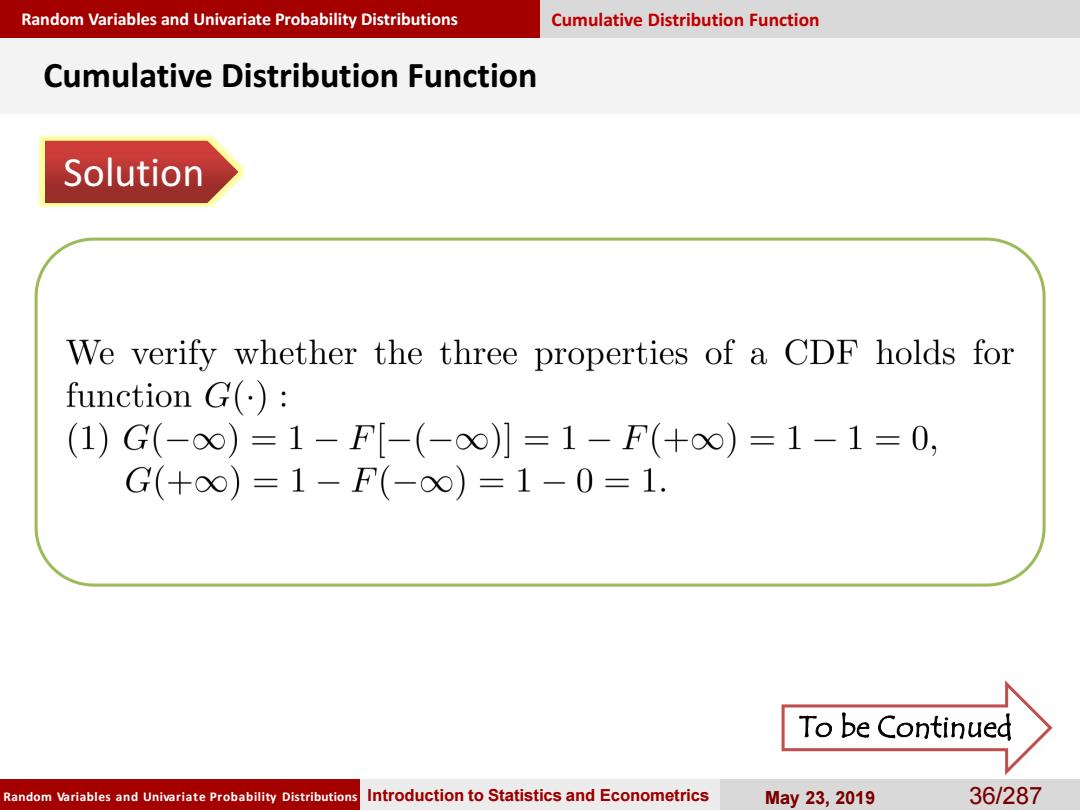
Random Variables and Univariate Probability Distributions Cumulative Distribution Function Cumulative Distribution Function Solution We verify whether the three properties of a CDF holds for function G(): (1)G(-0∞)=1-F[-(-0∞)川=1-F(+∞)=1-1=0, G(+0∞)=1-F(-0∞)=1-0=1. To be Continued Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May23,2019 36/287
Random Variables and Univariate Probability Distributions Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May 23, 2019 36/287 Cumulative Distribution Function Cumulative Distribution Function Solution To be Continued
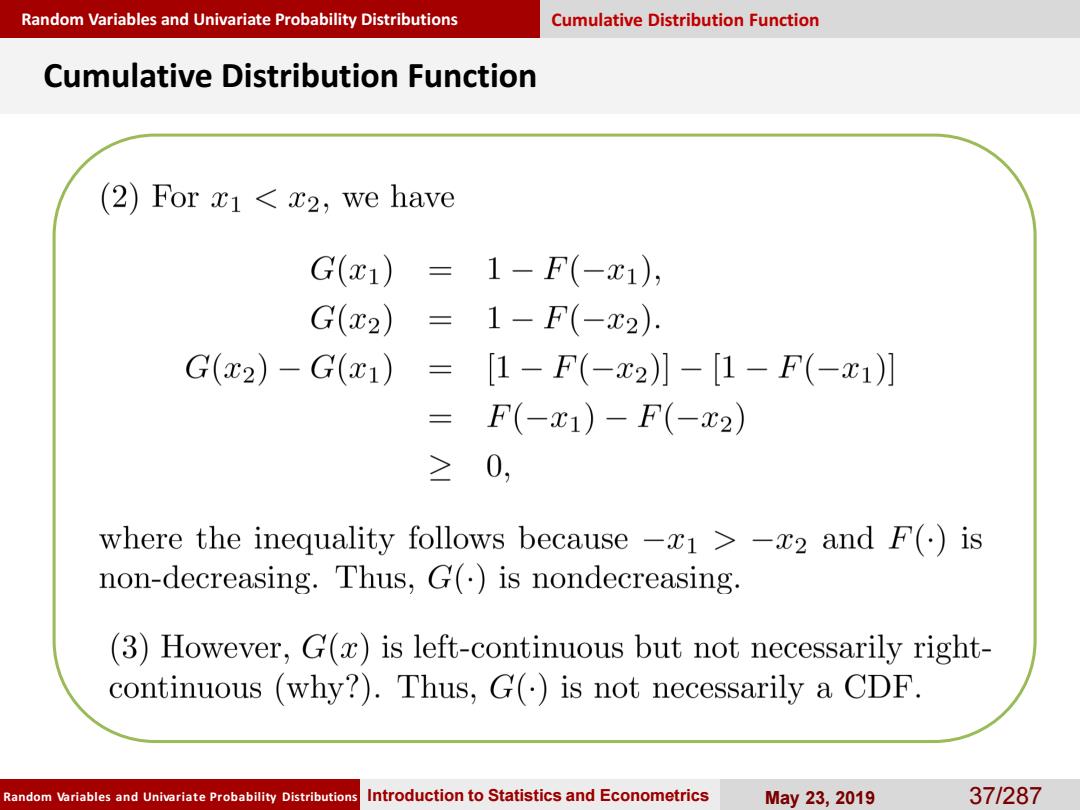
Random Variables and Univariate Probability Distributions Cumulative Distribution Function Cumulative Distribution Function (2)For z1 x2,we have G(c1)=1-F(-x1), G(x2)=1-F(-x2). G(x2)-G(x1)= [1-F(-x2)]-[1-F(-x1)] 三 F(-x1)-F(-x2) 0, where the inequality follows because-z1 >-z2 and F()is non-decreasing.Thus,G()is nondecreasing. (3)However,G(x)is left-continuous but not necessarily right- continuous (why?).Thus,G()is not necessarily a CDF. Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May23,2019 371287
Random Variables and Univariate Probability Distributions Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May 23, 2019 37/287 Cumulative Distribution Function Cumulative Distribution Function
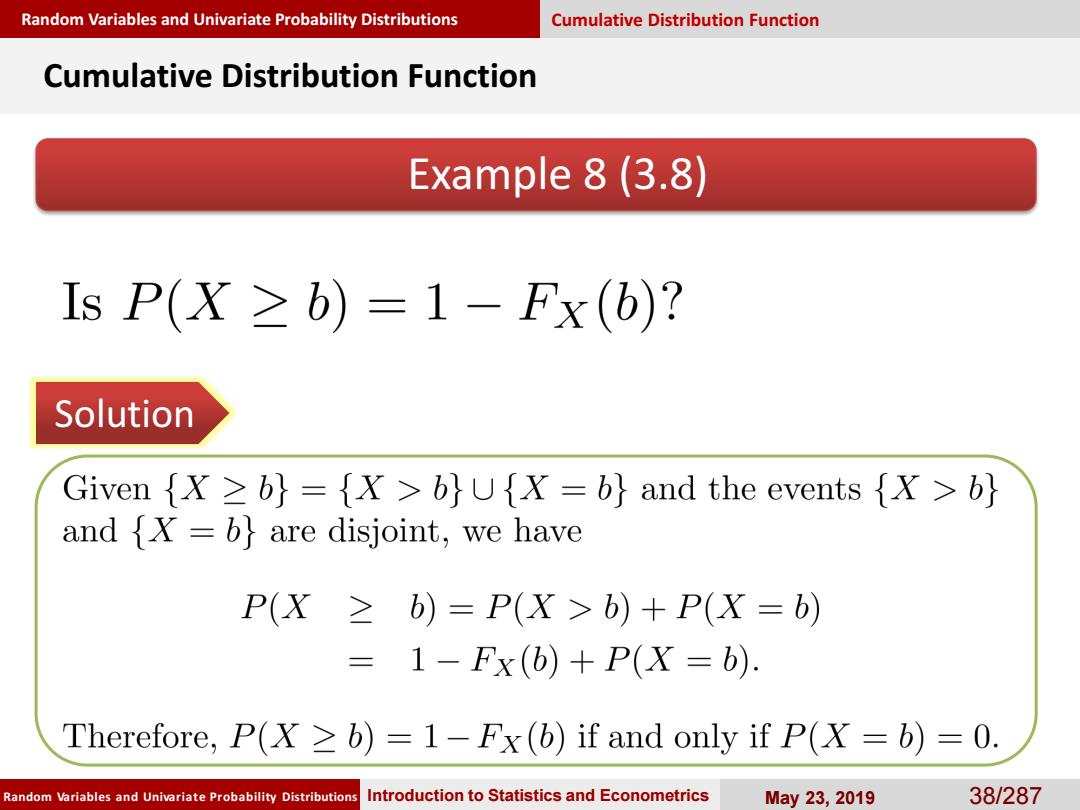
Random Variables and Univariate Probability Distributions Cumulative Distribution Function Cumulative Distribution Function Example 8 (3.8) IsP(X≥b)=1-Fx(b)? Solution Given{=>bu=b}and the events {X>b and {X =b}are disjoint,we have P(X 6)-P(X>6)+P(X=6) 1-Fx(b)+P(X=b): Therefore,P(X >b)=1-Fx(b)if and only if P(X=b)=0. Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May23,2019 38/287
Random Variables and Univariate Probability Distributions Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May 23, 2019 38/287 Example 8 (3.8) Cumulative Distribution Function Cumulative Distribution Function Solution

Random Variables and Univariate Probability Distributions Cumulative Distribution Function Cumulative Distribution Function Example 9(3.9).Mixture of Two Distributions Suppose F(x)and F2(x)are two CDF's.Is the linear combi- nation F(c)=pF(x)+(1-p)F2(c) a CDF?Here p is a constant. Solution The answer is yes,if 0<p<1.Here,the distribution F(x)is usually called a mixture of distributions F(x)and F2(x). Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May23,2019 39/287
Random Variables and Univariate Probability Distributions Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May 23, 2019 39/287 Example 9 (3.9). [ Mixture of Two Distributions ] Cumulative Distribution Function Cumulative Distribution Function Solution

Random Variables and Univariate Probability Distributions Cumulative Distribution Function Cumulative Distribution Function Remarks: A mixture of two distributions can provide a great deal of flexibility such as capturing skewness and heavy tails. One possibility for a mixed distribution to arise is that in an observed data,some observations are generated from one distribution,and the remaining observations are generated from another distribution. Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May23,2019 40/287
Random Variables and Univariate Probability Distributions Random Variables and Univariate Probability Distributions Introduction to Statistics and Econometrics May 23, 2019 40/287 Cumulative Distribution Function Cumulative Distribution Function Remarks: