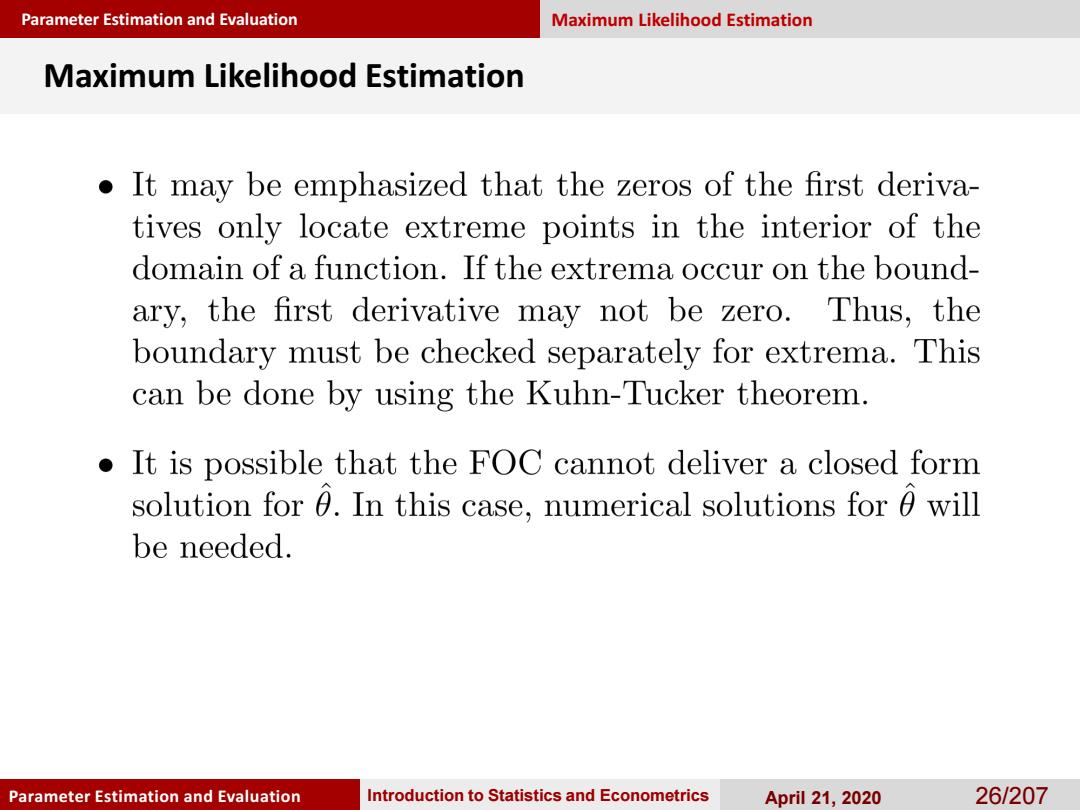
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation It may be emphasized that the zeros of the first deriva- tives only locate extreme points in the interior of the domain of a function.If the extrema occur on the bound- ary,the first derivative may not be zero.Thus,the boundary must be checked separately for extrema.This can be done by using the Kuhn-Tucker theorem. It is possible that the FOC cannot deliver a closed form solution for 0.In this case,numerical solutions for 0 will be needed. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 26/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 26/207 Maximum Likelihood Estimation Maximum Likelihood Estimation
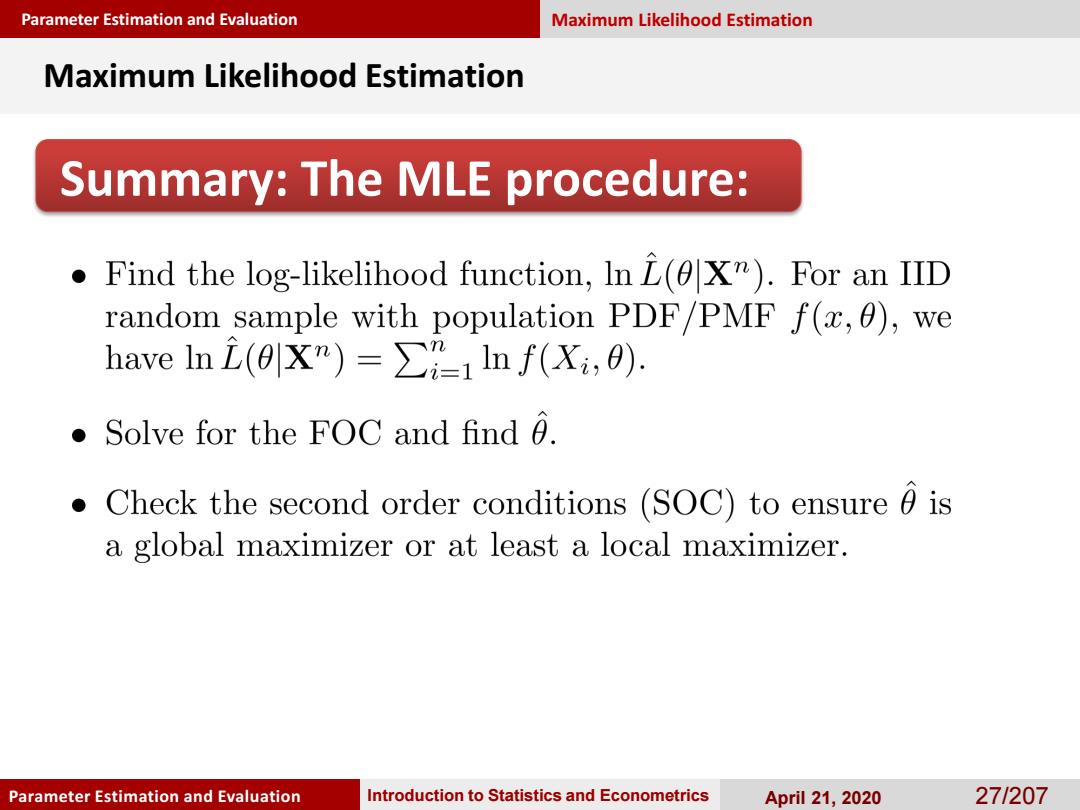
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Summary:The MLE procedure: Find the log-likelihood function,In L(X").For an IID random sample with population PDF/PMF f(x,0),we have In i(X")=>2 In f(X:,0). Solve for the FOC and find 0. Check the second order conditions(SOC)to ensure 0 is a global maximizer or at least a local maximizer. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 27/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 27/207 Maximum Likelihood Estimation Maximum Likelihood Estimation Summary: The MLE procedure:
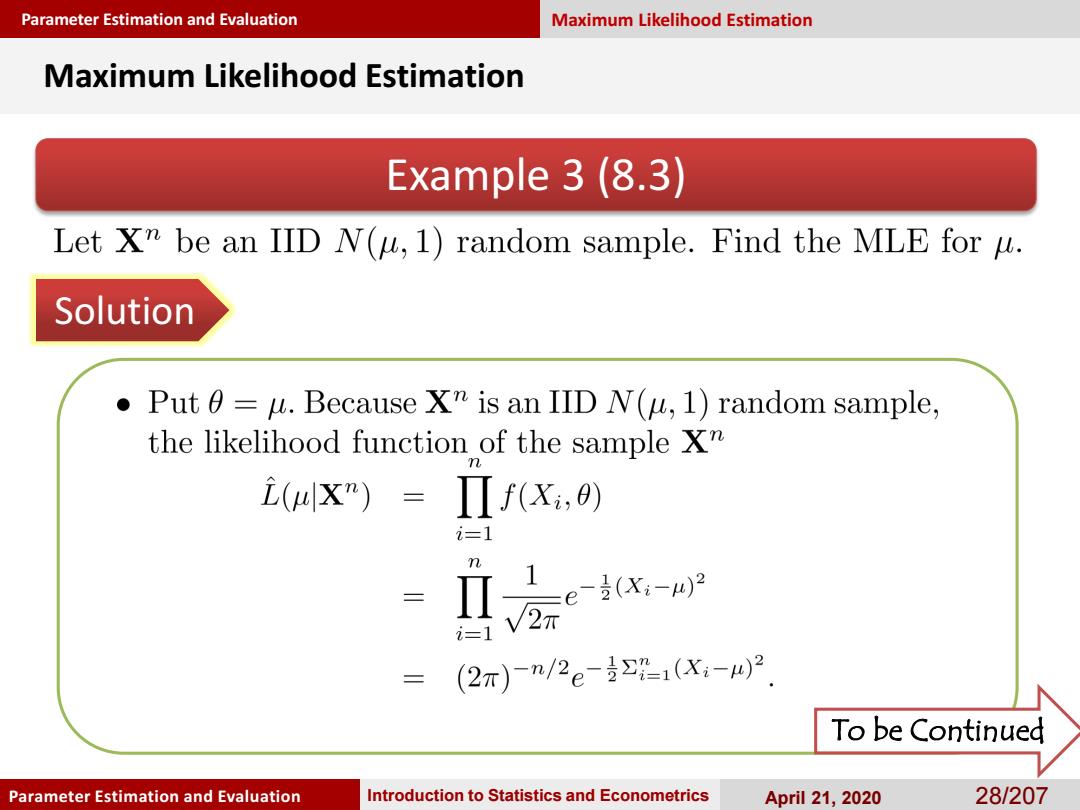
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Example 3 (8.3) Let X"be an IID N(u,1)random sample.Find the MLE for u. Solution Put a=u.Because X"is an IID N(u,1)random sample, the likelihood function of the sample X" i(4X")=Πf(X,0) i=1 ex- i=1 V2元 (2r)n/2e21(x:-w)2 To be Continued Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 28/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 28/207 Maximum Likelihood Estimation Maximum Likelihood Estimation Example 3 (8.3) Solution To be Continued

Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation It follows that the log-likelihood function m mi(ulx")=-2In(2)->(X:-)2. =1 ·The FOC is given by dIn (x") dIn i(ux") d du = 2 ∑(x:-)=0. 2=1 This gives the sample mean estimator =Xn. To be Continued Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 29/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 29/207 Maximum Likelihood Estimation Maximum Likelihood Estimation To be Continued
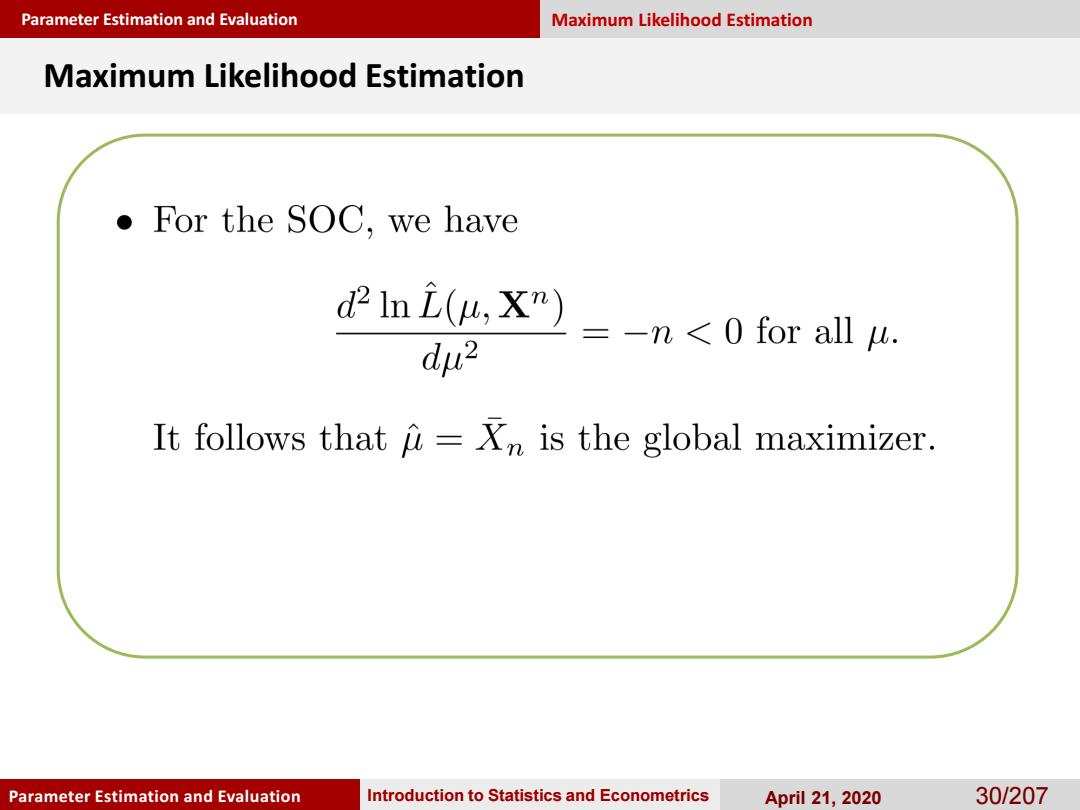
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation 。For the SOC,we have d2lni(μ,X") dμ2 =m<0 for all u. It follows that a=Xn is the global maximizer. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 30/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 30/207 Maximum Likelihood Estimation Maximum Likelihood Estimation