CONTENTS 8.1 Population and Distribution Model 8.2 Maximum Likelihood Estimation 8.3 Asymptotic Properties of MLE 8.4 Method of Moments and Generalized Method of Moments 8.5 Asymptotic Properties of GMM 8.6 Mean Squared Error Criterion 8.7 Best Unbiased Estimators 8.8 Cramer-Rao Lower Bound 8.9 Conclusion Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 16/207
Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 16/207 8.1 Population and Distribution Model 8.2 Maximum Likelihood Estimation 8.3 Asymptotic Properties of MLE 8.4 Method of Moments and Generalized Method of Moments 8.5 Asymptotic Properties of GMM 8.6 Mean Squared Error Criterion 8.7 Best Unbiased Estimators 8.8 Cramer-Rao Lower Bound 8.9 Conclusion CONTENTS

Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Question:How to estimate 0o based on a data set x"? R.Fisher proposed a general method of estimation called MLE.He demonstrated the advantage of this method by showing that it yields a sufficient estimator for parameter 0 whenever it exists and the MLE is asymptotically most efficient in terms of some sensible criterion Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 17/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 17/207 Maximum Likelihood Estimation Maximum Likelihood Estimation
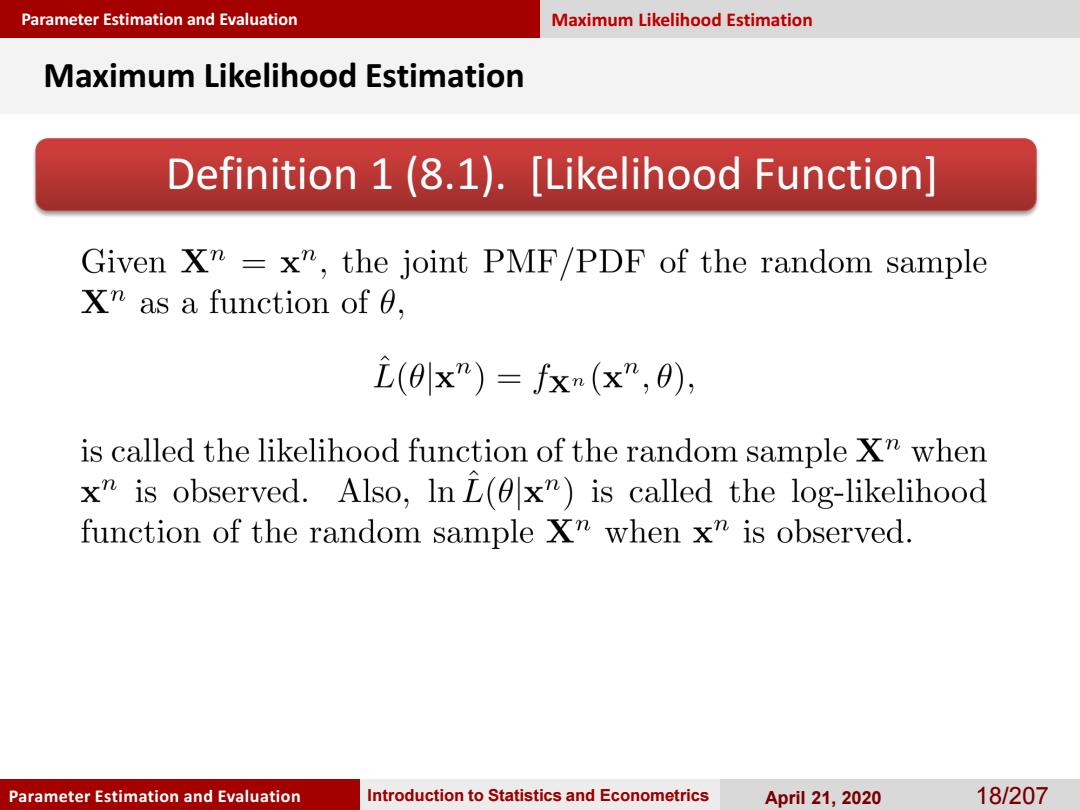
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Definition 1(8.1).[Likelihood Function] Given XT=x",the joint PMF PDF of the random sample Xm as a function of 0, (0xm)=fxn(x”,0), is called the likelihood function of the random sample X"when x"is observed.Also,In L(x")is called the log-likelihood function of the random sample Xm when x"is observed. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 18/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 18/207 Maximum Likelihood Estimation Maximum Likelihood Estimation Definition 1 (8.1). [Likelihood Function]

Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Remarks: The likelihood function L(x")is algebraically equal to the joint PDF or PMF of the random sample X"when Xn=xn. The conceptual difference between them is that the like- lihood L(x")is a function of 0,with x"held fixed. Given 0,the likelihood L(x")is a measure of the prob- ability or probability density with which the observed sample x"will occur. The joint PDF/PMF of the random sample X",fxm(x",0), is different from the population distribution f(x,0).The latter is the PDF/PMF of each random variable Xi. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 19/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 19/207 Maximum Likelihood Estimation Maximum Likelihood Estimation Remarks:
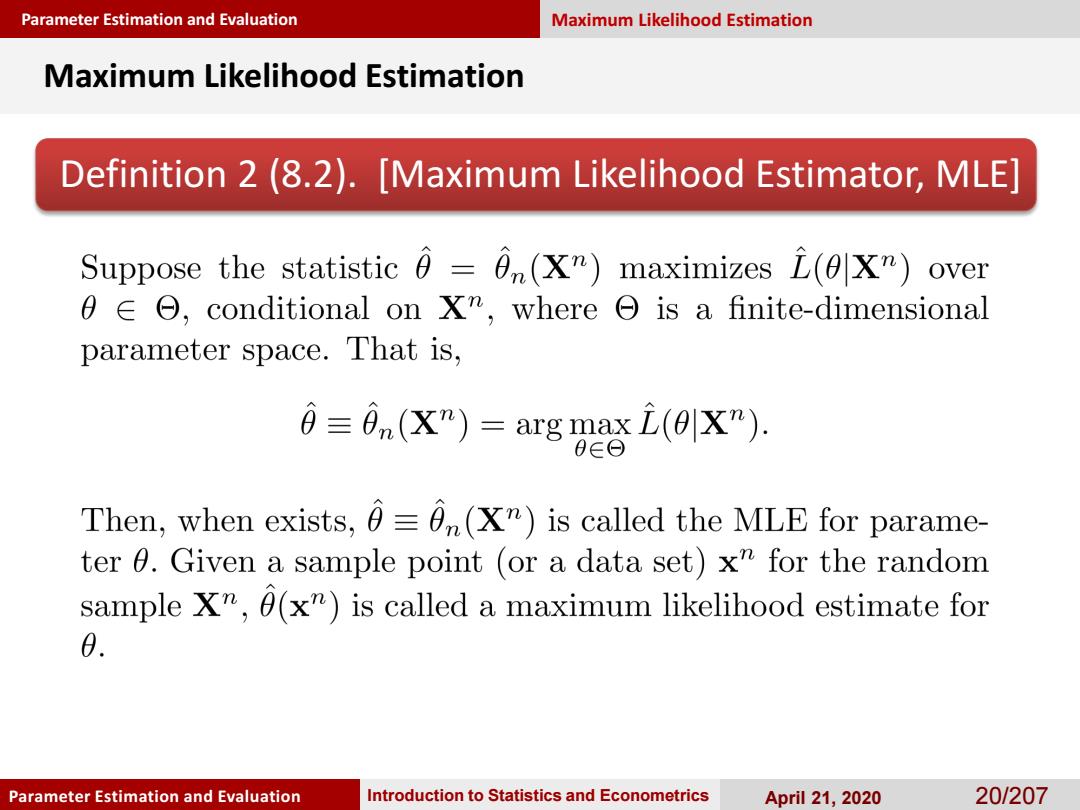
Parameter Estimation and Evaluation Maximum Likelihood Estimation Maximum Likelihood Estimation Definition 2(8.2).[Maximum Likelihood Estimator,MLE] Suppose the statistic 0=n(X")maximizes L(X")over 0∈曰,conditional on Xm,where曰is a finite-dimensional parameter space.That is, 9=0n(Xm)=arg maxL(0Xm). Then,when exists,=n(X")is called the MLE for parame- ter 0.Given a sample point (or a data set)x"for the random sample X",0(x")is called a maximum likelihood estimate for 0. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 20/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 20/207 Maximum Likelihood Estimation Maximum Likelihood Estimation Definition 2 (8.2). [Maximum Likelihood Estimator, MLE]